Scientific Tutor
Archive for the ‘Chemistry’ Category
Chem – Calorimetry
What is CALORIMETRY?
PAGE IS NOT COMPLETE
Chem – Heat of Fusion and Heat of Vaporization
What is the heat of fusion and heat of vaporization?
In the previous section of specific heat capacity we only discussed and did calculations for how energy affects substances within the same state (gas, liquid, solid) to change their temperature. However, in this section we will discuss how to use energy to cause chemical substances to go from one state to another (example: solid to liquid). This is called the heat of fusion or heat of vaporization.
Fusion = going between solid and liquid
Vaporization = going between liquid and gas
When you are either evaporating or condensing. The formula for energy and how it is involved with the heat of fusion or vaporization is below:
Heat Energy =
|
Mass * Heat of Fusion
|
Heat Energy =
|
Mass * Heat of Vaporization
|
| Q = | m * Δ H |
The letter Q represents heat energy (with units of J or cal), the letter m represents mass (with units of g), the symbol Δ H represents specific heat capacity (with units of J/g C or cal/g C). NOTICE that whether you are using heat of fusion or heat of vaporization the equation is the same. The only thing that changes is what column of the table you look at to obtain the number for heat of fusion or heat of vaporization. Those heat of fusion or heat of vaporization reference tables can be found here. This table also has melting and boiling points that will not be used in this section but will be used in a later section.
VIDEO Heat of Fusion or Vaporization Example 1: If 123g of H2O is boiled (from liquid to gas), how much heat energy is required? (Use this table for reference)
What information does the problem give you?
Answer:
Q = ?
m = 123g
Δ Hvap = 2260 J/g (vaporization because it says boiled)
What formula do we use to connect this information?
| Q = | m * Δ Hvap |
How do we fill in the formula with the information?
| Q = | 123g * 2260 J/g |
Calculate —-> (123 * 2260) = 277980
| Q = | 277980 J |
What is the complete answer?
COMPLETE ANSWER: 277980 J …..or….. 2.78 * 105 J
VIDEO Heat of Fusion or Vaporization Example 2: If 4kJ is released when freezing (from liquid to solid) CO2, what is the amount of mass of CO2 that is frozen? (Use this table for reference)
What information does the problem give you?
Answer:
Q = 4kJ —-> 4000 J
m = ?
Δ HFus = 2260 J/g (fusion because it says freezing)
What formula do we use to connect this information?
| Q = | m * Δ HFus |
How do we fill in the formula with the information?
| 4000 = | m * 184 |
How do we solve for m?
Answer: divide both sides by 184.
| 4000 = | m * 184 |
| 184 | 184 |
Cross out 184 on the right side
| 4000 = | m * 184 |
| 184 | 184 |
Simplify
| 4000 = | m |
| 184 |
Calculate —-> (4000 / 184) = 21.7
| 4000 = | 21.7 |
| 184 |
What is the complete answer?
COMPLETE ANSWER: 21.7 g
PRACTICE PROBLEMS: Calculate the missing information in the heat of fusion or vaporization equation. (Use this table for reference)
If 50g of H2O is frozen (from liquid to solid), how much heat energy is required?
Answer: 16700 J
If 42g of CO2 is evaporated (from liquid to gas), how much heat energy is required?
Answer: 24108 J
3200J is applied to melt H2O. What mass of H2O is melted?
Answer: 9.58g
If it takes 8600J to condense 700g of an unknown substance. What is the substance’s heat of vaporization?
Answer: 12.29 J/g
Chem – Specific Heat Capacity
What is specific heat capacity?
Energy or heat energy can also be involved in changing the temperature of a substance. Although it is not essential to know more about what temperature is for this section or lesson, you can review it in this link on temperature if you wish. The relationship between energy and a substance’s temperature is described by the equation below:
Heat Energy =
|
Mass * Specific Heat * Change in Temperature
|
| 1 | |
| Q = | m * c * Δ T |
The letter Q represents heat energy (with units of J or cal), the letter m represents mass (with units of g), the letter c represents specific heat capacity (with units of J/g C or cal/g C), and the symbol Δ T represents change in temperature (with units of C). Change in temperature means final temperature minus initial temperature (Tf – Ti).
The heat energy, mass, and temperature have all been described before. However, we have not yet discussed the specific heat capacity. It is actually a fancy scientific term for something that is very simple. The specific heat capacity is how much energy a certain substance can absorb. Therefore, each chemical substance has a specific heat capacity number that is unique to only that substance and each phase of that chemical substance. For example the specific heat capacity of liquid water will be different from the specific heat capacity of solid water (ice). In a typical chemistry class or book, they organize these specific heat capacities into a table. Most word problems will require you to look up the specific heat capacity on a table. Here is the link to that specific heat capacity table.
One trick that they can add to specific heat capacity problems is stating the amount of liquid H2O you have in milliliters instead of grams. Since 1 milliliter = 1 gram for liquid H2O you can just take however many milliliters they have and turn it into grams.
VIDEO Specific Heat Capacity Demonstrated Example 1: How much energy is required to heat 500g of liquid H2O from 22 C to 40 C. (Specific Heat Capacity Table).
What information does the problem give you?
Answer:
m = 500 g
Ti = 22 C
Tf = 40 C
c = 4.18 J/g C liquid H2O
What formula do we use to connect this information?
| Q = | m * c * Δ T |
How do we fill in the formula with the information?
| Q = | 500g * 4.18 J/g C * (40 C – 22 C) |
Simplify
| Q = | 500g * 4.18 J/g C * (18 C) |
Calculate
500 * 4.18 * 18 = 37620
COMPLETE ANSWER: 37620 J
VIDEO Specific Heat Capacity Demonstrated Example 2: If 34kJ energy is required to heat 620g of a solid substance from 24 C to 72 C what is the specific heat capacity of that substance? (Specific Heat Capacity Table).
What information does the problem give you?
Answer:
Q = 34 kJ —-> 34000 J
m = 620 g
Ti = 24 C
Tf = 72 C
c = ?
What formula do we use to connect this information?
| Q = | m * c * Δ T |
How do we fill in the formula with the information?
| 34000J = | 620g * c * (72C – 24C) |
Simplify
| 34000J = | 620g * c * (48C) |
How do we solve for c?
Answer: Divide both sides by 620g and 48C
| 34000J = | 620g * c * 48C |
| 620g * 48C | 620g * 48C |
Cross off 620g and 48C from the right side.
| 34000J = | 620g * c * 48C |
| 620g * 48C | 620g * 48C |
Simplify
| 34000J = | c |
| 620g * 48C | 1 |
How do I do the calculations?
Answer: 34000J / (620g * 48 C) = 1.14 J/g C
| 34000J = | 1.14 J/g C |
| 620g * 48C | 1 |
What is the complete answer?
COMPLETE ANSWER: 1.14 J/g C
VIDEO Specific Heat Capacity Demonstrated Example 3: If the temperature of 13ml of liquid H2O starts at 36 C and is heated with 650 J. What will be its final temperature? (Specific Heat Capacity Table).
What information does the problem give you?
Answer:
Q = 650 J
m = 13ml —-> 13g
Ti = 36 C
Tf = ?
c = 4.18 J/g C liquid H2O
What formula do we use to connect this information?
| Q = | m * c * Δ T |
How do we fill in the formula with the information?
| 650 = | 13 * 4.18 * (Tf – 36) |
Do not try to solve for Tf. Solve for (Tf – 36) first. To do that divide both sides by 13 and 4.18.
| 650 = | 13 * 4.18 * (Tf – 36) |
| 13 * 4.18 | 13 * 4.18 |
Cross off 13 * 4.18 from the right side.
| 650 = | 13 * 4.18 * (Tf – 36) |
| 13 * 4.18 | 13 * 4.18 |
Simplify
| 650 = | (Tf – 36) |
| 13 * 4.18 |
Calculate —-> 650 / (13 * 4.18) = 12
| 12 = | (Tf – 36) |
| 1 |
+ 36 to both sides
| 12 = | (Tf – 36) |
| + 36 | + 36 |
Cross off the 36 on the right side.
| 12 = | (Tf – 36) |
| + 36 | + 36 |
Simplify
| 12 = | Tf |
| + 36 |
Calculate —-> 12 + 36 = 48
| 48 = | Tf |
| 1 |
What is the complete answer?
COMPLETE ANSWER: Tf = 48 C
PRACTICE PROBLEMS: Calculate the missing information in the specific heat equation. Use the Specific Heat Capacity Table when needed.
How much energy is required to heat 80g of liquid H2O from 13 C to 57 C.
Answer: 14713 J
How much energy is required to heat 173g of solid iron from 205 C to 439 C.
Answer: 18217 J
If 6.4kJ energy is required to heat 510g of a solid substance from -12 C to 31 C what is the specific heat capacity of that substance?
Answer: 0.29 J/g C
If the temperature of 61ml of liquid H2O starts at 27 C and is heated with 840 J. What will be its final temperature?
Answer: 30.3 C
Chem – Hess’ Law
HESS’ LAW:
PAGE IS NOT YET COMPLETE
Hess’ Law is an idea that you can add or summarize multiple chemical equations together to learn about the overall enthalpy or energy information about them. However, this addition of equations is not the usual addition that you are used to. We have to manipulate the chemical equations and their enthalpy through multiplication, division, and reversal of signs (positive and negative). This concept is very hard to understand without intense study of the examples below.
Chem – Stoichiometry with Energy
HOW DO YOU USE STOICHIOMETRY WITH ENERGY?
You will probably want to have a strong foundation in the previous sections of…….before you learn about this section. If not go back and review those previous sections especially…… Stoichiometry with energy is simply using the different energy notations like you would a mole to mole ratio. To start we should look at a couple of different ways a chemical equation can be presented with energy. Then we can show how to write parts of that equation in a ratio.
The first most common way to see an equation with energy is like below.
200 kJ + N2(g) + 3 H2(g) <—-> 2 NH3(g)
This equation allows us to write ratios to the energy like:
What is the ratio of N2 to the energy?
| 1 N2 |
| 200 kJ |
or
| 200 kJ |
| 1 N2 |
What is the ratio of NH3 to the energy?
| 200 kJ |
| 2 NH3 |
or
| 2 NH3 |
| 200 kJ |
The second most common way to see an equation with energy is like below.
2 C4H10(l) + 13 O2(g) ——> 8 CO2(g) + 10 H2O (g) Δ H = -368 kJ/mol
This equation allows us to write ratios to the energy like:
What is the ratio of O2 to the energy?
| 13 O2 |
| 368 kJ |
or
| 368 kJ |
| 13 O2 |
What is the ratio of CO2 to the energy?
| 8 CO2 |
| 368 kJ |
or
| 368 kJ |
| 8 CO2 |
Notice the negative symbol of the energy does not appear anywhere in the ratios.
VIDEO Stoichiometry with Energy Conversions Demonstrated Example 1: How much energy is required to decompose 4 mol of MnI3?
78 kJ + 2 MnI3(s) ——> 3 I2(g) + Mn(s)
What information does the question supply us with?
Answer: 4 mol MnI3
What units does the question ask?
Answer: kJ
How do we set up the problem?
Answer:
| 4 mol MnI3 | kJ | |
| 1 |
What is the first conversion?
Answer: mole to kJ ratio
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 4 mol MnI3 | kJ = | kJ |
| mol MnI3 |
What is the next step?
Answer: Fill in the numbers and cross out units
| 4 mol MnI3 | 78 kJ = | kJ |
| 2 mol MnI3 |
Simplify
| 4 | 78 kJ = | kJ |
| 2 |
How do I do the calculations?
Answer: (4 * 78) / 2 = 156
What is the complete answer?
COMPLETE ANSWER: 156 kJ
VIDEO Stoichiometry with Energy Conversions Demonstrated Example 2: How many moles of Ca are created when 1400 kJ is released?
6 Ag(s) + Ca3(PO4)2(s) ——> 3 Ca(s) + 2 Ag3PO4(s) Δ H = -382 kJ/mol
What information does the question supply us with?
Answer: 1400kJ
What units does the question ask?
Answer: mole Ca
How do we set up the problem?
Answer:
| 1400 kJ | mole Ca | |
| 1 |
What is the first conversion?
Answer: mole to kJ ratio
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 1400 kJ | mole Ca = | mole Ca |
| kJ |
What is the next step?
Answer: Fill in the numbers and cross out units
| 1400 kJ | mole Ca = | mole Ca |
| kJ |
Simplify
| 1400 | 3 mole Ca = | mole Ca |
| 382 |
How do I do the calculations?
Answer: (1400 * 3) / 382 = 11
What is the complete answer?
COMPLETE ANSWER: 11 mole Ca
PRACTICE PROBLEMS: Calculate the energy or moles as needed.
How much energy is required to decompose 5 mol of N2O3?
32 kJ + 2 N2O3(g) ——> 2 N2(g) + 3 O2(g)
Answer: 80.0 kJ
How many moles of H2O are created when 97 kJ is released?
2 C2H6(l) + 7 O2(g) ——> 4 CO2(g) + 6 H2O(g) Δ H = -610 kJ/mol
Answer: 0.954 mole H2O
How many moles of CaCl2 are used up when 123 cal is released?
2 Na3PO4(aq) + 3 CaCl2(aq) ——> Ca3(PO4)2(s) + 6 NaCl(aq) + 74 cal
Answer: 4.99 mole CaCl2
How much energy is required to product 10.5 mole of C6H12O6?
6 C4(s) + 12 O2(g) + 24 H2(g) ——> 4 C6H12O6(s) Δ H = 78000 J/mol
Answer: 2.05 * 105 J
Chem – How to Calculate ΔH (Delta H) Part 3
HOW DO YOU CALCULATE ΔH (DELTA H)?
Once you memorized the relationship between the side the energy is on in a chemical equation, the Δ H and endothermic or exothermic, you are probably curious about how Δ H is calculated. It is calculated by the total energy contained in the molecules of products minus the total energy of the molecules in the reactants. Any time you are calculating the Δ of something it is always FINAL – INITIAL. Another way to say that is FINISH – START or PRODUCTS – REACTANTS. The formula is below.
Energy of products – Energy of reactants = Δ H
But how do we count the energy of the products or the energy of the reactants? It turns out that each chemical has an energy associated with it. Just like you would read the amount of calories off the back of a food label. You can also find tables where you can look up the energy of different chemicals. Where do we get the energy of the individual chemicals? Here is a link to the place where you find the enthalpy (energy) of each chemical. It is referred to in chemistry as the Δ Hf or the ENTHALPY OF FORMATION. REMEMBER to make sure the chemicals you look up also have the correct states of matter. If you want to find it in a chemistry text book, then it will usually be in the very back of the book in the index section.
VIDEO Calculate Δ H (DELTA H) Demonstrated Example 1: Use the balanced chemical equation below and calculate its Δ H. (Use this link look up the Δ Hf values)
CH4(g) + 2 O2(g) —-> CO2(g) + 2 H2O(g)
What is the energy of the molecules of the products?
Answer: CO2(g) = -394 kJ/mol H2O(g) = -242 kJ/mol
Do any of the molecules in the products have any coefficients?
Answer: Yes, H2O(g) 2 H2O(g) = 2 * (-242 kJ/mol)
What is the energy of the molecules of the reactants?
Answer: CH4(g) = -75 kJ/mol O2(g) = 0
Do any of the molecules in the reactants have any coefficients?
Answer: Yes, O2(g) 2 O2(g) = 2 * (0)
What is the general formula for Δ H?
Answer: Energy of products – Energy of reactants = Δ H
Modify the Δ H equation for this chemical equation.
(CO2(g) + 2 H2O(g)) – (CH4(g) + 2 O2(g)) = Δ H
Fill in the numbers for this specific equation.
(-394 + 2 * (-242)) – (-75 + 2 * (0)) = Δ H
Solve for Δ H
COMPLETE ANSWER: Δ H = -803 kJ/mol
VIDEO Calculate Δ H (DELTA H) Demonstrated Example 2: Use the Δ H and balanced chemical equation below and calculate the Δ Hf of H2Ba(s). (Use this link look up the Δ Hf values)
2 NaH(g) + BaCl2(s) —-> H2Ba(s) + 2 NaCl(s)Δ H = -536 kJ/mol
What is the energy of the molecules of the products?
Answer: H2Ba(s) = X kJ/mol NaCl(s) = -411 kJ/mol
Do any of the molecules in the products have any coefficients?
Answer: Yes, NaCl(s) 2 NaCl(s) = 2 * (-411 kJ/mol)
What is the energy of the molecules of the reactants?
Answer: NaH(g) = -55 kJ/mol BaCl2(s) = -858 kJ/mol
Do any of the molecules in the reactants have any coefficients?
Answer: Yes, NaH(g) 2 NaH(g) = 2 * (-55 kJ/mol)
What is the general formula for Δ H?
Answer: Energy of products – Energy of reactants = Δ H
Modify the Δ H equation for this chemical equation.
(H2Ba(s) + 2 NaCl(s)) – (2 NaH(g) + BaCl2(s)) = Δ H
Fill in the numbers for this specific equation.
( X + 2 * (-411)) – (2 * (-55) + -858) = -536
Solve for X
COMPLETE ANSWER: Δ Hf of H2Ba(s) = -682 kJ/mol
PRACTICE PROBLEMS: Calculate the Δ H or the Δ Hf as needed. (Use this link look up the Δ Hf)
Use the balanced chemical equation below and calculate its Δ H.
2 H2O(g) —-> 2 H2(g) + O2(g)
Answer: Δ H = +484 kJ/mol
Use the balanced chemical equation below and calculate its Δ H.
3 CaSO4(s) + 2 Al(OH)3(s) —-> 3 Ca(OH)2(s) + Al2(SO4)3(s)
Answer: Δ H = 462 kJ/mol
Use the Δ H and the balanced chemical equation below and calculate the Δ Hf of F–(g).
H+(g) + F–(g) —-> HF(g)Δ H = – 150 kJ/mol
Answer: Δ Hf of F–(g) = -120 kJ/mol
Use the Δ H and balanced chemical equation below and calculate the Δ Hf of CN(s).
2 HCN(g) + AgCN(s) —-> Ag(s) + H2(g) + 3 CN(s)Δ H = -113 kJ/mol
Answer: Δ Hf of CN(s) = 101 kJ/mol (do not forget to divide by the 3 coefficient)
Chem – Enthalpy and Enthalpy Change (ΔH) Part 2
WHAT IS ENTHALPY ΔH (DELTA H)?
Enthalpy is simply talking about energy in the form of heat. So ENTHALPY is HEAT ENERGY. Enthalpy is represented by Δ H (Delta H).
200kJ + N2(g) + 3 H2(g) —-> 2 NH3(g) Δ H = +200 kJ/mol
The above chemical reaction can be said to have a positive Δ H or is endothermic. All reactions with a positive Δ H are ENDOTHERMIC.
2 H2O(g) —-> 2 H2(g) + O2(g) + 147cal Δ H = -147 cal/mol
The above chemical reaction can said to have a negative Δ H or is exothermic. All reactions with a negative Δ H are EXOTHERMIC.
You should be able to use the information about Energy or Enthalpy to identify all representations of it in chemical reactions. Know what energy looks like in a reaction, how that relates to its Δ H, and how that relates to endothermic or exothermic.
This is a summary of what you have to know before you move on from this section:
| Endothermic |
| Energy absorbed |
| Energy appears on reactants |
| Δ H is positive |
AND
| Exothermic |
| Energy released |
| Energy appears on products |
| Δ H is negative |
PRACTICE PROBLEMS: Which side of the equation is the energy on? What is the Δ H? Is it endothermic or exothermic?
For the equation below, is the Δ H positive or negative? Is the reaction endothermic or exothermic?
C4(s) + 4 O2(g) —-> 4 CO2(g) + Energy
Answer: negative, exothermic
For the equation below, is the Δ H positive or negative? Is the reaction endothermic or exothermic?
330J + 2 Fe3+(aq) + 3 CO32-(aq) —-> Fe2(CO3)3(s)
Answer: +330J, endothermic
For the equation below, which side of equation is the energy? Is the reaction endothermic or exothermic?
H2SO4(aq) —–> H2(aq) + S(s) + 2 O2(aq)Δ H = positive
Answer: energy is on left side (reactants), endothermic
For the equation below, which side of equation is the energy? Is the reaction endothermic or exothermic?
2 H2O(g) —-> 2 H2(g) + O2(g)Δ H = -14kJ
Answer: right side (products) 14kJ, exothermic
Fill in the missing column sections with the information you are given. The first two lines are finished for you as an example. Highlight missing sections to reveal the answer once you have attempted.
| Side of Equation | Δ H | Endo/Exo |
| +45J in reactants | +45J | Endothermic |
| products | Negative | Exothermic |
| reactants | Positive | Endothermic |
| + 500KJ in products | -500KJ | Exothermic |
| + 300cal in reactants | +300cal | Endothermic |
| products | Negative | Exothermic |
Chem – How Energy is Represented in a Chemical Equation Part 1
HOW IS ENERGY REPRESENTED IN A CHEMICAL EQUATION?
Energy can be connected in different ways to a chemical equation. The first and easiest to recognize is when energy is presented in a chemical equation like the examples below.
200kJ + N2(g) + 3 H2(g) —-> 2 NH3(g)
Above the energy shown on the LEFT SIDE of the arrow (yield sign) by the number and units 200kJ. This is an example of a chemical reaction that absorbs energy. In chemistry they call this an ENDOTHERMIC (or ENDERGONIC) reaction.
2 H2O(g) —-> 2 H2(g) + O2(g) + 147cal
Above the energy shown on the RIGHT SIDE of the arrow (yield sign) by the number and units 147cal. This is an example of a chemical reaction that releases energy. In chemistry they call this an EXOTHERMIC (or EXERGONIC) reaction.
Chem – Conversion Between Joules and Calories
HOW DO YOU CONVERT BETWEEN JOULES AND CALORIES?
PAGE IS NOT YET COMPLETE
Chem – Energy
What is Energy?
Most sources will define energy as the ability to do work. However, you then have to define what work is. Usually following the definition route gets you nowhere. This definition is much easier to understand; Energy is the ability to move a mass (matter) against a resistance (Force). The easiest example of the use of energy is to pick an object like a book up off of a table. If you move the book up against gravity your muscles expend energy to do it. That movement of the book up means that positive energy (or work) is required. Another way to say it is that the book gained energy. The book gained what is called potential energy. You can now remove your hand from the book and it will fall. As it falls back to the table it releases that energy in the form of the crash of sound when it hits the table. If we call the table the zero point of our vertical picture then if the book is allowed to fall from the table to the floor it now requires negative energy (or does negative work) because the movement is down.
How do you think about energy in terms of chemicals and chemistry?
A molecule is held together by a bond. If you try and break it apart, you are trying to move a mass against a resistance. This is an example of positive energy use. When the atoms of certain elements come together (to form a compound) they are attracted to each other and therefore, use a negative amount of energy. Another way to say that is they release energy when they come together. So whether you are talking about moving atoms or moving books, they are both examples of how energy influences what happens.
What are the units of energy?
The units of energy are joules (J) or calories (cal). Joules are the SI unit or standard unit in science, however, calories are the more common term you will hear. When you talk about how many calories you burned during exercise you are talking about how much energy you expended. Very often in chemistry books, they will use kilojoules (kJ) or kilocalories, (kcal) so remember your metric conversions. The worst and most confusing unit that is sometimes inflicted upon students is Calories. Yes, it does not look any different then the original, but because of the capital C this means kilocalories.
Chem – LESSON 16: Thermochem and Energy
This is one of the most difficult lessons for most students. Make sure you put a lot of effort into this lesson.
NOTE: None of the thermochemical numbers for the Δ H of chemical equations are true (EXCEPT NUMBERS ON OR THAT COME FROM THE Δ H or Δ G or Δ S TABLES). Also when I say that a certain equation is endothermic or exothermic I assigned these distinctions to demonstrate an example. I never actually looked them up in a book to see if they were true to the chemical process you would see in a laboratory. The fact that I made them up does not make any of my methods for solving these examples incorrect. Just don’t take the number you see here to be absolute truth. All of the numbers are created to guide you through the process of how to solve these problems.
What is the lesson about?
This lesson is about how we define and use energy in chemical equations. It also gives descriptions on how to more abstract concepts like entropy, spontaneity, work, and how energy relates to things like pressure, work, and volume.
Why is it critical to understand?
Once you know about the concepts of energy surrounding an equation you can make predictions about how efficient a chemical reaction would be. The energy of the equations is also the building block to understand how quickly a chemical reaction will take place. Although the speed of a chemical will not be discussed directly until the Reaction Rates Lesson, many of the measurements on how to read reaction rate graphs are first discussed in this lesson. The real world applications of Thermodynamics include tasks like at what temperature should you cook meat to kill bacteria. You could also do calculations on how much energy is required to travel with your car over a certain distance and link that back to how much fuel you will need to carry.
What should you know before attempting this lesson?
If you have trouble in this lesson go back to sections on
New Learning Sections:
—> Converting Between Joules and Calories
—> How Energy is Represented in a Chemical Equation Part 1
—> How to Calculate Enthalpy (Delta H) Part 3
—> Hess’ Law
—> Specific Heat Capacity Part 1
—> Heat of Fusion and Heat of Vaporization Part 2
—> Entropy Definition and Relationship to Energy Part 1
—> Entropy and States of Matter Part 2
—> Calulating Δ n (Delta n) Part 3
—> Spontaneous / Spontaneity Definition
—> College: Calculating Delta G (first way)
—> Calculating Delta G (second way)
Reference Pages:
Worksheets:
Chem – LESSON 15: Acid / Base and pH
fadf
What should you know before attempting this lesson?
If you have trouble in this lesson go back to sections on
New Learning Sections:
—> Definitions of Acids and Bases
—> College: Further Definition of Acids and Bases
—> Naming Acids (Acid Nomenclature)
—> Strong Versus Weak Acids and Bases
—> Conjugate Acid and Conjugate Base
—> Neutralization Reactions
—> pH and pOH calcuations
Reference Pages:
Worksheets:
Chem – Forming Precipitation Products in a Chemical Equation and Labeling the Precipitant
What skills do I need before I start predicting the products of a precipitation reaction?
Forming the products of a precipitation reaction requires bringing together a lot of different previous skills you have learned in your chemistry class or on this website. The primary sections you need to know or should review are how to form ionic compounds and how to break apart ionic compounds and balancing chemical equations and types of chemical equations and precipitation definition and precipitation rules (solubility table). The skills in those previous sections will be talked about in detail with the examples in this section but they will be much more brief.
How do you form the products of a precipitation reaction (precipitation equation)?
Typically precipitation reactions are double displacement (double replacement) types of reactions with ionic compounds. Which means you need to rearrange the two ionic compounds in the reactants to from two new ionic compounds in the products. The information you are given is like the example below.
Predict the products of the chemical reaction (chemical equation), indicate the precipitant, and balance the equation.
Na3PO4(aq) + CaCl2(aq) ——>
Answer: The precipitant is Ca3(PO4)2
2 Na3PO4(aq) + 3 CaCl2(aq) ——> Ca3(PO4)2(s) + 6 NaCl(aq)
Examples: Predict the products of the chemical reaction (chemical equation), indicate the precipitant, and balance the equation. Make sure to use the solubility table and precipitation definitions if you need them. Another useful tool is the ion periodic table. VIDEO Demonstration of Forming Products of a Precipitation Reaction Examples 1.
Na2S(aq) + AgNO3(aq) ——>
Answer: The precipitant is Ag2S
Na2S(aq) + 2 AgNO3(aq) ——> Ag2S(s) + 2 NaNO3(aq)
ZnBr2(aq) + (NH4)3PO4(aq) ——>
Answer: The precipitant is Ni3(PO4)2
3 ZnBr2(aq) + 2 (NH4)3PO4(aq) ——> Zn3(PO4)2(s) + 6 NH4Br(aq)
Ba(OH)(aq) + VCl3(aq) ——>
Answer: The precipitant is V(OH)3
3 Ba(OH)2(aq) + 2 VCl3(aq) ——> 3 BaCl2(aq) + 2 V(OH)3(s)
PRACTICE PROBLEMS: Predict the products of the chemical reaction (chemical equation), indicate the precipitant, and balance the equation. Make sure to use the solubility table and precipitation definitions if you need them. Another useful tool is the ion periodic table.
Chem – Precipitation Rules (Solubility Table)
What are the precipitation rules (what is the solubility table)?
In a word question or chemical equation you will not always be shown whether a chemical is SOLUBLE (aq) or INSOLUBLE (s). Sometimes you will have to determine that for yourself. For this reason we turn to what they call the PRECIPITATION RULES or the SOLUBILITY TABLE. It is the simplest way to visualize, organize, and memorize the precipitation rules.
I also created an expanded solubility table that includes more ions you might see and work with in class. Typically they are only seen in AP and college courses and sometimes not even then.
MEMORIZATION TRICK: In AP or college classes you may have to memorize the solubility table. If you only memorize the ions that are soluble, you only have to memorize half or less of the solubility table. DO NOT focus on the exceptions. Memorize the general ions first.
Make sure before you try to understand the examples and practice problems below that you know how to break apart and put together ionic compounds. If you have any questions on ionic compounds refer to the sections on how to form ionic compounds and how to break apart ionic compounds. In the examples I will point out and use the RARE exceptions in the solubility table. However, with practice problems and future use of these ideas I WILL NOT require the RARE exceptions to be known for the solubility table.
Examples: Determine if the chemical is INSOLUBLE or SOLUBLE and give what state it would be marked with in a chemical equation (aq or s). Make sure to use the solubility table and precipitation definitions if you need them. Another useful tool is the ion periodic table. VIDEO Solubility Table Examples 1.
| Chemical | Answer |
| NaNO3 | Soluble (aq) |
| RuPO4 | Insoluble (s) |
| Mn3(SO4)2 | Soluble (aq) |
| V(OH)2 | Insoluble (s) |
| (NH4)2CO3 | Soluble (aq) |
| Li3PO4 | Soluble (aq) |
| AgCl | Insoluble (s) |
| CaS | Soluble (aq) |
PRACTICE PROBLEMS: Determine if the chemical is INSOLUBLE or SOLUBLE and give what state it would be marked with in a chemical equation (aq or s). Make sure to use the solubility table and precipitation definitions if you need them. Another useful tool is the ion periodic table.
| Chemical | Answer |
| MoI | Soluble (aq) |
| CaSO4 | Soluble (aq) |
| Zr3(PO4)2 | Insoluble (s) |
| W(CO3)2 | Insoluble (s) |
| NH4OH | Soluble (aq) |
| Fe2S3 | Insoluble (s) |
| Cs2CO3 | Soluble (aq) |
| FrOH | Soluble (aq) |
Chem – Precipitation Definition and Chemical Equations
What is Precipitation?
It is the act of one substance changing state and therefore removing itself from the solution / mixture.
The change of state will happen when you get a change of conditions (either a change of temperature, pressure, or having chemicals added to it). The most common way you see precipitation is when water molecules in the gas state collect into clouds and then change to liquid water in the form of rain. Your local weather person will always talk about the chance for precipitation. They mean the chance it will rain or fog or cause dew to form on your car. In the case of water in the air the process can also be called condensation, which we talked about in a previous lesson.
For the purposes of chemistry classes we do not usually talk about precipitation happening in the form of rain. Instead we often talk about two different aqueous solutions coming together in one container and how those two solutions may cause solid compounds or molecules to form. This solid compound or molecule that forms can also be referred to as the precipitant. An example of how this precipitant is written in a balanced chemical equation is given below:
6 KOH(aq) + Fe2(SO4)3(aq) –—-> 2 Fe(OH)3(s) + 3 K2SO4(aq)
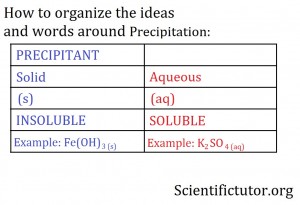
The example means that one solution containing KOH in one cup and another solution containing Fe2(SO4)3 in a completely separate cup are poured together into a container to create the solid precipitate Fe(OH)3 and an aqueous solution of K2SO4 . In this section, you want to be able use definitions to describe the different parts and states in the chemicals equations. The words you will be using to describe the chemical equations are PRECIPITANT, SOLID, INSOLUBLE, AQUEOUS, and SOLUBLE. I have arranged how I want you to think about these words in a small table below. All the words or symbols in BLUE mean the same and all the words or symbols in RED mean the opposite of the blue.
Examples: Determine which chemicals are the PRECIPITANT which are INSOLUBLE, and which are SOLUBLE in the balanced chemical equations below. VIDEO Precipitant Examples 1.
2 Na3PO4(aq) + 3 CaCl2(aq) ——> Ca3(PO4)2(s) + 6 NaCl(aq)
Answer: The PRECIPITANT and INSOLUBLE chemical is Ca3(PO4)2 . The SOLUBLE chemicals are NaCl and Na3PO4 and CaCl2 .
3 MgSO3(aq) + 2 K3PO4(aq) ——> 3 K2(SO3)(aq) + Mg3(PO4)2(s)
Answer: The PRECIPITANT and INSOLUBLE chemical is Mg3(PO4)2 . The SOLUBLE chemicals are MgSO3 and K3PO4 and K2(SO3) .
PRACTICE PROBLEMS: Determine which chemicals are the PRECIPITANT which are INSOLUBLE, and which are SOLUBLE in the balanced chemical equations below. Use the solubility picture if needed.
CaCl2(aq) + Rb2CO3(aq) ——> CaCO3(s) + 2 RbCl(aq)
Answer: The PRECIPITANT and INSOLUBLE chemical is CaCO3 . The SOLUBLE chemicals are CaCl2 and Rb2CO3 and RbCl .
2 CrBr3(aq) + 3 (NH4)2S(aq) ——> 6 NH4Br(aq) + Cr2S3(s)
Answer: The PRECIPITANT and INSOLUBLE chemical is Cr2S3 . The SOLUBLE chemicals are CrBr3 and (NH4)2S and NH4Br .
Sr(OH)2(aq) + 2 HgC2H3O2(aq) ——> 2 HgOH(s) + Sr(C2H3O2)2(aq)
Answer: The PRECIPITANT and INSOLUBLE chemical is HgOH . The SOLUBLE chemicals are Sr(OH)2 and HgC2H3O2 and Sr(C2H3O2)2 .
Chem – Boiling Point Elevation and Freezing Point Depression
What are freezing point depression and boiling point elevation?
Freezing point depression and boiling point elevation are talking about how you take a solution and influence it to change its freezing point (freezing temperature) or boiling point (boiling temperature). The freezing point and the boiling point change when you add more of either the solute or the solvent to the solution therefore, changing it’s molality. The vast majority of the time this happens you will cause the freezing point to decrease (depress) and the boiling point to increase (elevate). Books and teachers usually talk about this in terms of adding or changing only the solute because it is more simple to explain if only one of the factors is changing.
Where is freezing point depression and boiling point elevation used in every day life?
Most people tend to use these concepts to improve their lives at least once a week. If you are cooking noodles and you want them to cook faster, then you throw some salt in the water that you are boiling and this raises (elevates) the boiling point of the water and therefore the noodles cook faster. If you live in a cold climate, then when it snows trucks come around to spread salt on the roads. This salt mixes with the ice or water and causes the freezing point to decrease (depress) and therefore the ice can more easily turn back into the liquid form of water and run off the street to decrease slips and falls and car accidents.
What is the formula for freezing point depression and boiling point elevation?
The formula for freezing point depression and boiling point elevation is below.
| Δ T = | m * k * i |
| 1 |
Delta T ( Δ T ) represents the change in temperature with units of degrees Celsius. Little m represents molality with units of mols per kg (mol / kg). The k represents the constant for the change in freezing point (kf) or the constant for the change in boiling point (kb). Each chemical you deal with has a different kf or kb and you only use the kf or kb of the chemical that is the solvent. For this learning section I am only going to use the kf and kb of water (H2O) since the most common solvent that most teachers use especially in high school. Finally we come to the I which represents the ionization constant that we discussed in the learning section before this one. Let us check out the examples below.
For water:
Kf = -1.86 C kg / mol
Kb = 0.512 C kg / mol
VIDEO Freezing Point Depression and Boiling Point Elevation Demonstrated Example 1: If you put 2 moles of NaBr into 200 kg of water, then what will be your change in freezing point? Kf = -1.86 C kg / mol
Step 1: Gather information
mol of NaBr = 2 moles
mass of water = 200kg
Kf = -1.86 C kg / mol
i is related to NaBr
i = ?
m = ?
Δ T = ?
Step 2: which equation connects my information?
Δ T = m * k * i
Step 3: solve for i
Since i is related to the chemical NaBr then when we put NaBr in water it breaks up since it is ionic. It breaks up into two ions Na+1 and Br-1.
Answer: i = 2
Step 4: solve for m
The m or molality = mol solute / kg of solvent.
| m = | mol solute |
| kg solvent |
Step 5:
| m = | 2 mol NaBr |
| 200 kg water |
Step 6: calculate
| m = | 2 mol |
| 200 kg |
Answer: m = 0.01 mol / kg
Step 7: solve for Δ T
| Δ T = | m * k * i |
| 1 |
Step 8: put in numbers and units
| Δ T = | 0.01 mol * -1.86 C kg * 2 |
| kg mol |
Step 9: cross out units
| Δ T = | 0.01 mol * -1.86 C kg * 2 |
| kg mol |
Step 10: Simplify
| Δ T = | 0.01* -1.86 C * 2 |
| 1 |
Step 11: calculate
0.01* -1.86 * 2 = – 0.0372
COMPLETE ANSWER: Δ T = – 0.0372
VIDEO Freezing Point Depression and Boiling Point Elevation Demonstrated Example 2: If the change in temperature for the boiling point is 2.1 C with 500 L of water then how many moles of NF3 did you put into the water? Kb = 0.512 C kg / mol
Step 1: Gather information
Δ T = 2.1 C
mass of water = 500 L = 500 kg
Kb = 0.512 C kg / mol
entire formula is Δ T = m * k * i
i is related to NF3
i = ?
m = ?
mols of NF3 = ?
Step 2: which equation connects my information?
Δ T = m * k * i
Step 3: solve for i
Since i is related to the chemical NF3 then when we put NF3 in water it does not break up since it is covalent. Therefore, it remains as one molecule.
Answer: i = 1
Step 4: solve for m
| Δ T = | m * k * i |
| 1 | 11 |
Step 5: put in numbers and units
| 2.1 C = | m * 0.512 C kg / mol * 1 |
| 1 |
Step 6: divide both sides by the Kb and the i
| 2.1 C = | m * 0.512 C kg / mol * 1 |
| 0.512 C kg / mol * 1 | 0.512 C kg / mol * 1 |
Step 7: cross out the matching stuff on the right side.
| 2.1 C = | m * 0.512 C kg / mol * 1 |
| 0.512 C kg / mol * 1 | 0.512 C kg / mol * 1 |
Step 8: simplify
The m or molality = mol solute / kg of solvent.
| 2.1 C = | m |
| 0.512 C kg / mol * 1 |
Step 9: calculate
2.1 C / ( 0.512 C kg / mol * 1 ) = 4.10
m = 4.10 mol / kg
Step 10: solve for the moles of NF3
The m or molality = mol solute / kg of solvent.
| m = | mol solute |
| kg solvent |
Step 11: fill in the numbers and units
| 4.10 mol / kg = | mol solute |
| 500 kg |
Step 12: multiply both sides by 500 kg
| 500 kg * 4.10 mol / kg = | mol solute * 500 kg |
| 500 kg |
Step 13: cross out the 500kg on the right side
| 500 kg * 4.10 mol / kg = | mol solute * 500 kg |
| 500 kg |
Step 14: simplify
| 500 kg * 4.10 mol / kg = | mol solute |
| 1 |
Step 15: calculate
500 * 4.10 = 2050
COMPLETE ANSWER: moles of NF3 = 2050 moles
PRACTICE PROBLEMS: Solve the freezing point depression and boiling point elevation problems below. Try to use a regular periodic table. However if you need you can use your metal / non-metal periodic table and your ion periodic table. Remember the constants for water…….Kf = -1.86 C kg / mol……and..….Kb = 0.512 C kg / mol
If you put 3.0 moles of LiBr into 150 kg of water what will be your change in boiling point?
Answer: Δ T = 0.020 C
If you put 5.0 moles of Br2 into 120kg of water what will be the change in freezing point?
Answer: Δ T = – 0.078 C
QUESTION SOLVING for molality
How many moles of CaF2 will it take to change the boiling temperature of a solution with 305 L of water by 1.3 degrees C?
Answer: 260 moles
If an unknown covalent chemical changes the freezing temperature of a solution of 40 kg of water by -0.60 C then how many moles of that covalent chemical were added?
Answer: 12.9 moles
Chem – The Ionization Constant
What is the ionization constant?
The ionization constant is a factor you have to take into account when you talk about splitting apart chemicals. Mostly, the ionization constant has to do with splitting apart ionic compounds. It is estimated by the number of ions you get after you break apart a chemical. While in truth, it is a little more complex than that we will go into. We will not go into that complexity here.
What is the ionization constant used for?
Most often you will see the ionization constant used with the equations having to do with the freezing point depression and boiling point elevation. Since those two concepts will be explained in the next sections, I will not go into them here. All that matters is that they relate to the formula below.
| Δ T = | m * k * i |
| 1 |
This is the equation for freezing point depression and boiling point elevation, but we only need to know one component of it for now. Forget about all the rest of the letters and representations. All we are going to focus on is the I. The I is the ionization constant. Do not worry about the formula because we are NOT going to use it. If you remember way back to your compounds and bonding or maybe your nomenclature lessons, there was a way you learned to take apart ionic chemical compounds. We are going to use the same skills here, but add another layer of complexity to it. Check out the Examples below.
VIDEO Ionization Constant Demonstrated Example 1: If you have the chemical compound CH4 what is the ionization constant when you put it in water?
Step 1:
What kind of chemical is CH4, ionic or covalent?
Answer: It is covalent (made up of all non-metals)
Step 2:
If it is covalent what happens to it when it encounters the water? Does it break up?
Answer: No because water is polar.
Step 3:
So when you put one CH4 in how many molecules / particles / atoms do you get out?
Answer: 1
Step 4:
So what is the ionization constant of CH4?
COMPLETE ANSWER: 1 = ionization constant
VIDEO Ionization Constant Demonstrated Example 2: If you have the chemical compound NaBr what is the ionization constant when you put it in water?
Step 1:
What kind of chemical is NaBr, ionic or covalent?
Answer: It is ionic (made up of metals and non-metals)
Step 2:
If it is ionic, what happens to it when it encounters the water? Does it break up?
Answer: Yes because water is polar.
Step 3:
So when you put one NaBr in how many molecules / particles / atoms / ions do you get out?
Answer: 2 (one Na+1 and one Br1-)
Step 4:
So what is the ionization constant of NaBr?
COMPLETE ANSWER: 2 = ionization constant
VIDEO Ionization Constant Demonstrated Example 3: If you have the chemical compound CaF2 what is the ionization constant when you put it in water?
Step 1:
What kind of chemical is CaF2, ionic or covalent?
Answer: It is ionic (made up of metals and non-metals)
Step 2:
If it is ionic what happens to it when it encounters the water? Does it break up?
Answer: Yes because water is polar.
Step 3:
So when you put one CaF2 in how many molecules / particles / atoms / ions do you get out?
Answer: 3 (one Ca+2 and two F–)
Step 4:
So what is the ionization constant of CaF2?
COMPLETE ANSWER: 3 = ionization constant
VIDEO Ionization Constant Demonstrated Example 4: If you have the chemical compound BaSO4 what is the ionization constant when you put it in water?
Step 1:
What kind of chemical is BaSO4, ionic or covalent?
Answer: It is ionic (made up of metals and non-metals)
Step 2:
If it is ionic what happens to it when it encounters the water? Does it break up?
Answer: Yes because water is polar.
Step 3:
So when you put one BaSO4 in how many molecules / particles / atoms / ions do you get out?
Answer: 2 (one Ba+2 and one SO42-)
Step 4:
So what is the ionization constant of BaSO4?
COMPLETE ANSWER: 2 = ionization constant
PRACTICE PROBLEMS: Determine what the ionization constant is for the chemicals below. Try to use a regular periodic table. However if you need you can use your metal / non-metal periodic table and your ion periodic table.
| Chemical | Answer |
| CO2 | 1 |
| RbI | 2 |
| BaF2 | 3 |
| Li2O | 3 |
| FrOH | 2 |
| Ca(NO3)2 | 3 |
| Ra3P2 | 5 |
| Cs2CO3 | 3 |
| SiH4 | 4 |
Chem – Molarity
What is Molarity?
Molarity is by far the most common way to measure a solution concentration. So whenever you see the word concentration in a problem always immediately think molarity. The definition of molarity is moles of solute per liters of solution. I have written the equation below.
| Molarity = | Moles of Solute |
| Liters of Solution |
In order to think of it in a more simplistic way and help with problem solving I have simplified the formula into just units like below.
| Molarity = | Moles |
| Liters |
You can further shorten this into the letter representations of the units.
| M = | mol |
| L |
Capital M represents molarity. The mol represents moles. Capital L represents liters. When you see the M in a sentence it should usually be next to a number like 5 M. That means 5 molarity and it is a unit.
Examples: Solve for the molarity problems below.
If you put 0.4 mols of a solute in a beaker and then fill up with water to 3 L. What is your molarity?
Answer: 0.13 M
A 9M solution was made by filling a container up with 7L of solution. How many moles did it take?
Answer: 63 mol
If you used 2.8 moles to make a 6.3 M solution. What is the volume of that solution?
Answer: 0.44 L
VIDEO Molarity Demonstrated Example 1: A 15M solution was made by filling a container up with 4.2L of solution. How many moles did it take?
What information are we given?
Answer:
Molarity = 15M
Volume = 4.2L
What does the question ask for?
Answer: moles = ?
How do we set up the problem?
Answer: Start with the equation
| M = | mol |
| L |
What can we fill in for the equation?
Answer: The information we are given
| 15 M = | mol |
| 4.2 L |
How do we solve?
Multiply both sides by 4.2 L
| 4.2 L * 15 M = | mol * 4.2 L |
| 4.2 L |
Cross out 4.2 L on the right side
| 4.2 L * 15 M = | mol * 4.2 L |
| 4.2 L |
Simplify
| 4.2 L * 15 M = | mol |
| 11 |
Multiply the left side
| 4.2 L * 15 M = | mol |
| 11 |
Result
| 63 = | mol |
| 11 |
COMPLETE ANSWER: 63 mol
VIDEO Molarity Demonstrated Example 2: If you used 7.6 moles of BaCl2 to make a 1.2 M solution. What is the volume of that solution?
What information are we given?
Answer:
moles = 7.6 mol
molarity = 1.2 M
The BaCl2 does not matter.
What does the question ask for?
Answer: Volume = ? L
How do we set up the problem?
Answer: Start with the equation
| M = | mol |
| L |
What can we fill in for the equation?
Answer: The information we are given
| 1.2 M = | 7.6 mol |
| L |
How do we solve?
Multiply both sides by L
| L * 1.2 M = | 7.6 mol * L |
| L |
Cross out L on the right side
| L * 1.2 M = | 7.6 mol * L |
| L |
Simplify
| L * 1.2 M = | 7.6 mol |
| 1 |
Divide both sides by 1.2 M
| L * 1.2 M = | 7.6 mol |
| 1.2 M | 1.2 M |
Cross out 1.2 M on the left side
| L * 1.2 M = | 7.6 mol |
| 1.2 M | 1.2 M |
Simplify
| L = | 7.6 mol |
| 1.2 M |
Divide the right side
| L = | 6.3 |
| 11 |
COMPLETE ANSWER: 6.3 L
PRACTICE PROBLEMS: Solve for the MOLARITY problems below.
If you put 4.7 mols of a solute in a beaker and then fill up with water to 8.6 L. What is your molarity?
Answer: 0.55 M
A 0.58 M solution was made by filling a container up with 1.3 L of solution. How many moles did it take?
Answer: 0.75 mol
If you used 3.4 moles to make a 23 M solution. What is the volume of that solution?
Answer: 0.15 L
If you used 6.5 moles to make a 4.2 M solution. What is the volume of that solution in mL?
Answer: 155 mL
Chem – Mass to Volume Conversion of Water
A special consideration for this lesson is that since the metric system was designed around the use of water as a standard for measurement a milliliter of water is the same as a gram of water. It is also the same as a liter of water equals a kilogram of water. THIS ONLY FOR WATER ON THE SURFACE OF THE EARTH!!!
1 mL = 1 g
or
1 L = 1 kg
Although you will not encounter this in your chemistry class, it is also good to keep in mind that this conversion for water only works at or near sea level on earth. You cannot use this conversion outside of earth’s surface.
This section also uses some material from the units prefixes and unit conversions sections. Please go back and review them if you are have trouble with this section.
Examples: Solve for the water conversion problems below. VIDEO Mass to Volume Examples 1.
If you have 95 mL of water how many grams of water is that?
Answer: 95g
How many liters of water are required to provide 62 kilograms of water?
Answer: 62 L
73 kilograms of water on the surface of the earth translates to how many milliliters?
Answer: 73,000 mL
PRACTICE PROBLEMS: Solve for the water conversion problems below.
If you have 0.23 mL of water how many grams of water is that?
Answer: 0.23g
How many liters of water are required to provide 39 kilograms of water?
Answer: 39 L
8.4 kilograms of water on the surface of the earth translates to how many milliliters?
Answer: 8400 mL
How many kilograms is 120000 mL of water?
Answer: 120 kg
Chem – Concentration and Saturation
What is concentration?
Concentration is a way to define the amount of a substance in a certain amount of space. Luckily concentration is a natural thing for people to measure. Our sense of taste is how we measure concentration. Since every bite we take is approximately the same size (volume) then all that we measure when we taste something sweet is the amount of sugar in the space of our mouth.
What is saturation?
The other terminology that you have to get used to in this lesson are words about how much solute you put in the solvent. The three words we have to understand are unsaturated, saturated, and supersaturated. These three words come about because different amounts of solute in a solvent can change what you observe happening in the container. I like to think about the amount of solute you can put into a solvent as a party in a house. The house can only hold so many people. Just like the solvent can only hold so much solute. You can allow a small number of people into the house, just like you can put a small amount of solute into the solvent (unsaturated). You can fill the house to maximum capacity, just like you can put the maximum amount of solute into the solvent (saturated). If you want to go beyond that point of maximum then you have to change the conditions. In a house party you would then have to open up the back yard to allow more people in than can fit in your house. In a solution you can do a similar thing by changing the temperature or pressure to allow more solute into the solution (supersaturated).
Chem – Solutions and Solubility Definitions
What is a solution?
Unfortunately there are many definitions you have to learn in the solution lesson before you can move on any further. If you do not learn them first they will be handicapped the rest of the lesson. First we will focus on the definition of a solution. A solution is when two or more substances are mixed together on a molecular level. Different parts of a solution are not able to be separated simply by waiting for them to fall out. You have to change some kind of condition to separate them, like temperature or pressure. We also want to know what a solution is in terms of a mathematical perspective. A solution is A SOLUTE + A SOLVENT. Now that may seem confusing at first but really a solute is just one substance like salt and a solvent is another substance like water. It actually does not matter which one you call the solute and which one you call the solvent, but most of the time the teacher will either name which one is which or they will tend to call the solid (like salt) the solute and the liquid (like water) the solvent. In fact, in most classes you will only talk about the solute as a solid and the solvent as a liquid.
What is solubility?
Next we have to make a clear definitions for soluble and insoluble. Soluble is when two or more substances are able to mix together their individual molecules. For example, if you mix sugar in your tea then the sugar breaks up and blends in with the tea. You can say that the sugar is soluble in the tea. Insoluble is when two or more substances are not able to mix together their individual molecules. For example, if you mix a granite rock with water. The rock will not break up and blend in with the water so the granite rock is insoluble in water. How well different substances mix together we refer to as solubility. We can say a substance has high or low solubility in another. For example, butter has high solubility in olive oil but butter has low solubility in water. Solubility usually refers to a situation of a solid chemical mixing in a liquid chemical but the state of each chemical really does not matter. You can have two gases mix and still call them soluble in each other. For example oxygen gas (O2) and has high solubility in nitrogen gas (N2).
What is Miscible?
As if we did not have enough definitions already, we can now come to miscible versus immiscible. They are the exact same definition as soluble versus insoluble. That is miscible is the same thing as soluble while immiscible is the same thing as insoluble. Why chemistry has two definitions for the same thing may not make a whole lot of sense but miscible and immiscible usually refer to two liquids mixing. For example, olive oil is immiscible in water.
What kind of things are mixtures but not solutions?
Finally we come to the mixtures of things that do not interact with each other on the individual molecule scale but instead as large chunks of thousands of the same molecules that are stuck together. These are known as colloids or suspensions. The easiest way to tell colloids and suspensions apart from solutions is that colloids or suspensions if left to sit in a container (while at the same temperature) will separate into different substances after hours or days and will therefore form layers in the container. However, solutions will never separate out just by allowing them to sit in a container (while at the same temperature). Colloids are any clumps of molecules that are up to 1 micrometer (um) in size. Suspensions are clumps of molecules that are lager than 1 micrometer (um) in size. A classic suspension example is soil (dirt) in water. When you first mix the soil and water together they looked like they will stay mixed forever but as soon as you stop mixing you will see the clumps of soil start to float to the bottom and separate out.
Tyndyll?
Chem – LESSON 14: Solutions
What is the lesson about?
Solutions are a mixture of two or more things. In this lesson, you will learn about how chemistry defines mixtures, how it measures those mixtures, and how those mixtures behave as something different from each of their individual components. We live in a world of solutions and so this lesson helps you define how to analyze the solutions around you.
Why is it critical to understand?
Most of the lesson hinges on two sections. First, understand the definition of a solution. Second, focus on the ability to calculate and understand molarity. Molarity becomes useful for later lessons and that is especially true for AP and college students.
What should you know before attempting this lesson?
If you have trouble in this lesson go back to sections on Equations, Solving for an Unknown, Unit Conversions, Calculating the Molar Mass of Compounds, Definition and Layout of a Chemical Equation, States of Matter in a Chemical Equation, Balancing Chemical Equations Part 1, Tips and Tricks for Balancing Equations Part 2, Types of Chemical Equations, Prediction types of Chemical Equations Part 1, , Grams to Moles Conversions and Combining Stoichiometry and Molar Mass.
New Learning Sections:
—> Solution and Solubility Definitions
—> Concentration and Saturation
—> Mass to Volume Conversion of Water
—> Molarity (Molar Concentration)
—> Dilutions
—> Molality
—> The Ionization Constant Part 1
—> Boiling Point Elevation and Freezing Point Depression Part 2
—> Solving for Change in Temperature of a Solution Part 3
—> College: The Ionization Constant and Molality Part 4
—> Precipitation Definition and Precipitation with Chemical Equations Part 1
—> Precipitation Rules (Solubility Table) Part 2
—> Forming Precipitation Products in a Chemical Equation and Labeling the Precipitant Part 3
—> Complete Ionic Equation Part 4
—> Net Ionic Equations (Spectator Ions) Part 5
Reference Pages:
Worksheets:
Chem – Effusion
What is Effusion?
Effusion and Diffusion are very similar. Diffusion is the movement of particles or molecules across one or more barriers with tiny holes in it. Effusion is a simplified type of Diffusion. That is, effusion is the movement of particles or molecules through only one hole between only two containers. Here is a video demonstration. Regardless, you do not really have to know any of that to succeed in this section. The equation for how fast (the rate) that effusion happens is below. The equation is what is important.
| Rate of gas 1 = | Square root of Molar Mass of gas 2 |
| Rate of gas 2 | Square root of Molar Mass of gas 1 |
Or short hand
| Rate of gas 1 = | Sqrt MM gas 2 |
| Rate of gas 2 | Sqrt MM gas 1 |
Sqrt stands for SQUARE ROOT OF. MM stands for MOLAR MASS OF.This formula says that the rate of effusion or diffusion of a gas depends on the square root of its molar mass. That suggests that lighter gas effuse or diffuse slightly faster. These are not absolute rates. Meaning they are not always the same rate for a particular gas in any conditions. Rather they are relative rates. They are only a comparison of these two particular gases at these particular conditions. It is like saying a Lamborghini travels twice as fast as a Ford. In that comparison of cars I did not actually say how fast each of the cars were going. Instead, I compared them to one another. This formula is doing the same thing to gasses. If you try the numbers in the formula it says that He gas travels or effuses at twice the rate of CH4 gas. We will try this exact problem later as a demonstrated example. Be careful with this formula. Many people are fooled into writing this formula incorrectly or plugging in the numbers in the wrong spot because how the formula is written does not match how they are thinking of it. This will become clearer in the demonstrated examples.
Examples: Solve for the following effusion problems. Use this periodic table link to look up the molar masses.
If the rate of effusion of H2 gas is 15. What is the rate of effusion of N2 gas?
Answer: 3.96
If you have an unknown gas that effuses at a rate 2 times that of F2 what is the molar mass of that gas?
Answer: 9.5 g/mol
How much faster is the rate of effusion of O2 gas to that of CO2 gas?
Answer: 1.17 times faster
VIDEO Effusion Demonstrated Example 1: If the rate of effusion of Ne gas is 5. What is the rate of effusion of Cl2 gas? Use this periodic table link to look up the molar masses.
Step 1:
What information are we given?
Answer:
Rate of Ne = 5
(Look up) Molar Mass Ne = 20 g/mol
(Look up) Molar Mass Cl2 = 71 g/mol
Step 2:
What does the question ask for?
Answer: Rate of Cl2 = ?
Step 3:
How do we set up the problem?
Answer: Start with the equation
| Rate of Ne = | Sqrt MM Cl2 |
| Rate of Cl2 | Sqrt MM Ne |
Step 4:
What can we fill in for the equation?
Answer: The information we are given (red).
| 5 = | Sqrt 71 |
| Rate of Cl2 | Sqrt 20 |
Step 5:
How do we rearrange the equation?
Answer: Multiply both sides by Rate of Cl2(red).
| Rate of Cl2 (5) = | Sqrt 71 * Rate of Cl2 |
| Rate of Cl2 | Sqrt 20 |
Step 6:
Cross out Rate of Cl2 on the left side.
| Rate of Cl2(5) = | Sqrt 71 * Rate of Cl2 |
| Rate of Cl2 | Sqrt 20 |
Step 7:
Simplify
| (5) = | Sqrt 71 * Rate of Cl2 |
| Sqrt 20 |
Step 8:
How do we rearrange the equation?
Answer: Multiply both sides by Sqrt 20 and Divide by Sqrt 71 (red).
| Sqrt 20 (5) = | Sqrt 71 * Rate of Cl2 * Sqrt 20 |
| Sqrt 71 | Sqrt 20 * Sqrt 71 |
Step 9:
Cross out Sqrt 20 and Sqrt 71 on left
| Sqrt 20 *(5) = | Sqrt 71 * Rate of Cl2 * Sqrt 20 |
| Sqrt 71 | Sqrt 71 * Sqrt 20 |
Step 10:
Simplify
| Sqrt 20 * (5) = | Rate of Cl2 |
| Sqrt 71 |
Step 11:
How do I do the calculations?
Answer: ((Sqrt 20) * 5) / (Sqrt 71) = 2.65
| Sqrt 20 * (5) = | 2.65 |
| Sqrt 71 |
Step 12:
What is the complete answer?
COMPLETE ANSWER: 2.65
VIDEO Effusion Demonstrated Example 2: If you have an unknown gas that effuses at a rate 3 times that of Br2 what is the molar mass of that gas? Use this periodic table link to look up the molar masses.
Step 1:
What information are we given?
Answer:
Rate of Unknown = 3
Rate of Br2 = 1
(Look up) Molar Mass of Br2 = 160 g/mol
Step 2:
What does the question ask for?
Answer: Molar Mass of Unknown = ?
Step 3:
How do we set up the problem?
Answer: Start with the equation
| Rate of Unkno = | Sqrt MM Br2 |
| Rate of Br2 | Sqrt MM Unkno |
Step 4:
What can we fill in for the equation?
Answer: The information we are given (red).
| 3 = | Sqrt 160 |
| 1 | Sqrt MM Unkno |
Step 5:
How do we rearrange the equation?
Answer: Multiply both sides by Sqrt MM Unknown (red).
| 3 * Sqrt MM Unkno = | Sqrt 160 * Sqrt MM Unkno |
| 1 | Sqrt MM Unknown |
Step 6:
Cross out Sqrt MM Unkown on right side.
| 3 * Sqrt MM Unkno = | Sqrt 160 * Sqrt MM Unkno |
| 1 | Sqrt MM Unknown |
Step 7:
Simplify
| 3 * Sqrt MM Unkno = | Sqrt 160 |
| 1 | 1 |
Step 8:
How do we rearrange the equation?
Answer: Divide both sides by 3 (red).
| 3 * Sqrt MM Unkno = | Sqrt 160 |
| 1 * 3 | 1 * 3 |
Step 9:
Cross out 3 on left side.
| 3 * Sqrt MM Unkno = | Sqrt 160 |
| 1 * 3 | 1 * 3 |
Step 10:
Simplify
| Sqrt MM Unkno = | Sqrt 160 |
| 1 | 3 |
Step 11:
How do I do the calculations to help further simplify?
Answer: Sqrt 160 / 3 = 4.216
| Sqrt MM Unkno = | 4.216 |
Step 12:
Square both sides causing the elimination of the square root on the left.
| (Sqrt MM Unkno)2 = | (4.216)2 |
Step 13:
Simplify
| MM Unkno = | (4.216)2 |
Step 14:
How do I do the calculations?
Answer : (4.216)2 = 17.78
Step 15:
What is the complete answer?
COMPLETE ANSWER: 17.78 g/mol
VIDEO Effusion Demonstrated Example 3: How much faster is the rate of effusion of He gas to that of CH4 gas? Use this periodic table link to look up the molar masses.
Step 1:
What information are we given?
Answer:
(Look up) Molar Mass He = 4 g/mol
(Look up) Molar Mass CH4 = 16 g/mol
Step 2:
What does the question ask for?
Answer: Because this is a ratio we can solve for both.
Rate of CH4 = ?
Rate of He = ?
Step 3:
How do we set up the problem?
Answer: Start with the equation
| Rate of He = | Sqrt MM CH4 |
| Rate of CH4 | Sqrt MM He |
Step 4:
What can we fill in for the equation?
Answer: The information we are given (red).
| Rate of He = | Sqrt 16 |
| Rate of CH4 | Sqrt 4 |
Step 5:
How do we simplify the math?
Answer: Apply the square roots (sqrt)
sqrt 16 becomes 4……..sqrt 4 becomes 2
| Rate of He = | 4 |
| Rate of CH4 | 2 |
Step 6:
How do I do the calculations?
Answer: 4 / 2 = 2
| Rate of He = | 2 |
| Rate of CH4 | 1 |
Step 7:
What is the complete answer?
COMPLETE ANSWER: Helium is twice the rate of CH4……or 2 to 1
PRACTICE PROBLEMS: Solve these effusion problems. Use this periodic table link to look up the molar masses.
If the rate of effusion of N2 gas is 20. What is the rate of effusion of F2 gas?
Answer: 17.17
If you have an unknown gas that effuses at a rate 2.3 times that of Cl2 what is the molar mass of that gas?
Answer: 13.42
How much faster is the rate of effusion of H2S gas to that of Ar gas?
Answer: 1.08 times faster
Now try the gas laws worksheets
Chem – Partial Pressures
What are Partial Pressures?
The concept behind partial pressures is quite simple, but many books and example questions make the thinking behind them overly complicated. Partial pressures is all about adding up the pressures of each gas separately in a container to get the overall (total) gas pressure inside that container. For example, our atmosphere is a container. 99% of our atmosphere is made up for Nitrogen (N2), Oxygen (O2), and Carbon Dioxide (CO2). At sea level the pressure of the N2 is about 0.7 atm, the pressure of the O2 is about 0.2 atm, and the pressure of CO2 is about 0.1 atm. If you add all those up you get 1 atm which is the total pressure of our atmosphere at sea level. So all the gas pressures together equals the total.
N2 + O2 + CO2 = Total Pressure
We use an example like this above to extrapolate a more complex equation like the one below.
P1 + P2 + P3…… = PT
P1 is the pressure of gas 1, P2 is the pressure of gas 2, P3 is the pressure of gas 3, the dot dot dot (…) just means we could keep adding more pressures if more gasses were in the container. PT is pressure total. In theory, this equation could have just a P1 through P2 or it could have P1 through P1000000. It all depends on how many gasses that you have in that particular container.
Examples: Solve for the following partial pressure problems.
If a container has 4 atm of He and 7atm of Ar what is the total pressure?
Answer: 11 atm
If a container has 6 atm of F2, 2 atm of N2 and a total pressure of 12 atm, how much pressure is the Ne exerting?
Answer: 4 atm
Cl2 is bubbled through water and collected in an inverted test tube that is immersed in the same water. If the pressure of the Cl2 bubbled into the test tube is 1.5 atm and the total pressure in the test tube is 1.8 atm then what is the pressure of the H2O gas in the tube.
Answer: 0.3 atm
VIDEO Partial Pressure Demonstrated Example 1: If a container has 9 atm of Ne, 1 atm of He and a total pressure of 18 atm. What is the partial pressure of the Ar in the containter?
Step 1:
What information are we given?
Answer:
Ne pressure = 9 atm
He pressure = 1 atm
Total pressure = 18 atm
Step 2:
What does the question ask for?
Answer : Ar pressure = ?
Step 3:
How do we set up the problem?
Answer: Start with the equation
| PNe + PHe + PAr = | PT |
Step 4:
What can we fill in for the equation?
Answer: The information we are given (red).
| 9 + 1 + PAr = | 18 |
Step 5:
How do we rearrange the equation?
Answer: Minus 9 and 1 from the left side and right side (red).
| 9 + 1 + PAr – 9 – 1 = | 18 – 9 – 1 |
Step 6:
Simplify
| PAr = | 18 – 9 – 1 |
Step 7:
How do I do the calculations?
Answer: 18 – 9 – 1 = 8
Step 8:
What is the complete answer?
COMPLETE ANSWER: 8 atm
VIDEO Partial Pressure Demonstrated Example 2: N2 is bubbled through water and collected in an inverted test tube that is immersed in the same water. If the pressure of the N2 bubbled into the test tube is 2.3 atm and the total pressure in the test tube is 2.7 atm then what is the pressure of the H2O gas in the tube.
Step 1:
What information are we given?
Answer:
N2 pressure = 2.3 atm
Total pressure = 2.7 atm
Step 2:
What does the question ask for?
Answer : H2O pressure = ?
Step 3:
How do we set up the problem?
Answer: Start with the equation
| PN2 + PH2O = | PT |
Step 4:
What can we fill in for the equation?
Answer: The information we are given (red).
| 2.3 + PH2O = | 2.7 |
Step 5:
How do we rearrange the equation?
Answer: Minus 2.3 from the left side and right side (red).
| 2.3 + PH2O – 2.3 = | 2.7 – 2.3 |
Step 6:
Simplify
| PH2O = | 2.7 – 2.3 |
Step 7:
How do I do the calculations?
Answer: 2.7 – 2.3 = 0.4
Step 8:
What is the complete answer?
COMPLETE ANSWER: 0.4 atm
PRACTICE PROBLEMS: Solve these partial pressure problems below.
If a container has 3.5 atm of O2 and 1.2 atm of N2 what is the total pressure?
Answer: 4.7 atm
If a container has 5 atm of Cl2, 6 atm of BH3 and a total pressure of 13 atm, how much pressure is the Ar exerting?
Answer: 2 atm
CH4 is bubbled through water and collected in an inverted test tube that is immersed in the same water. If the pressure of the CH4 bubbled into the test tube is 2.8 atm and the total pressure in the test tube is 3.2 atm then what is the pressure of the H2O gas in the tube.
Answer: 0.4 atm
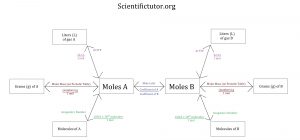
Chem – Gas Stoichiometry
What is gas stoichiometry?
In this section you are going to put to use a lot of the previous information you have learned in this lesson. You are also going to combine the information from the gas laws lesson with other sections like calculating the molar mass of compounds and combining stoichiometry and molar mass conversions. Make sure you have gone over or know these past sections before you enter this section. One new item that we need to understand in this chapter is how we treat STP conditions when we are doing stoichiometry. If you use the ideal gas law equation at STP conditions and calculate how many liters one mole will produce you come out with 22.4 L. So we can use this as a ratio for gas stoichiometry. I have demonstrated it below.
At STP this is a ratio you can use:
| 22.4 L |
| 1 mol |
We can now fit this into our new conversions map for gas stoichiometry and use it in the gas stoichiometry problems below.
VIDEO Gas Stoichiometry Demonstrated Example 1: At STP 3 L of H2 gas can make how many moles of NH3?
N2(g) + 3 H2(g) —-> 2 NH3(g)
Step 1:
What information does the question supply us with?
Answer: 3 L H2
Step 2:
What units does the question ask?
Answer: mol NH3
Step 3:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 2 arrows when we go from Liters of A —> moles of A —> moles of B. 2 arrows = 2 conversions
Step 4:
How do we set up the problem?
Answer:
| 3 L of H2 | mol NH3 | ||
| 1 |
Step 5:
What is the first conversion?
Answer: STP ratio (liters to mole ratio)
Step 6:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 3 L of H2 | mol | mol NH3 | |
| L |
Step 7:
What is the next step?
Answer: Fill in the numbers and cross out units
| 3 L of H2 | 1 mol | mol NH3 | |
| 22.4 L |
Step 8:
Simplifiy
| 3 H2 | 1 mol | mol NH3 | |
| 22.4 |
Step 9:
What is the next conversion?
Answer: mole to mole ratio (coefficient ratio)
Step 10:
How do I set it up?
Answer: units first, set up the units that you need to cancel out (in red)
| 3 H2 | 1 mol | NH3 = | mol NH3 |
| 22.4 | H2 |
Step 11:
What is the next step?
Answer: Fill in the numbers and cross out units
| 3 H2 | 1 mol | 2 NH3 = | mol NH3 |
| 22.4 | 3 H2 |
Step 12:
Simplify
| 3 | 1 mol | 2 NH3 = | mol NH3 |
| 22.4 | 3 |
Step 13:
How do I know I am done with conversions?
Answer: The only units left are the units that match the answer. In this case mol and NH3
| 3 | 1 mol | 2 NH3 = | mol NH3 |
| 22.4 | 3 |
Step 14:
How do I do the calculations?
Answer: (3 * 2) / (22.4 * 3) = 0.89
| 3 | 1 mol | 2 NH3 = | 0.89 mol NH3 |
| 22.4 | 3 |
COMPLETE ANSWER: 0.089 mol NH3
VIDEO Gas Stoichiometry Demonstrated Example 2: How many Liters of O2 gas at STP can be made from a sample of 18 grams of H2O? You will need a periodic table to help solve this problem.
2 C6H11OH(l) + 17 O2(g) ——> 12 CO2(g) + 12 H2O(g)
Step 1:
What information does the question supply us with?
Answer: 18 g H2O
Step 2:
What units does the question ask?
Answer: L O2
Step 3:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 3 arrows when we go from Grams of A —> moles of A —> moles of B —> Liters of B. 3 arrows = 3 conversions
Step 4:
How do we set up the problem?
Answer:
| 18 g H2O | L O2 | |||
| 1 |
Step 5:
What is the first conversion?
Answer: molar mass (grams to mole ratio) of H2O found on the periodic table
Step 6:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 18 g H2O | mol | L O2 | ||
| g |
Step 7:
What is the next step?
Answer: Fill in the numbers and cross out units
| 18 g H2O | 1 mol | L O2 | ||
| 18 g |
Step 8:
Simplify
| 18 H2O | 1 mol | L O2 | ||
| 18 |
Step 9:
What is the next conversion?
Answer: mole to mole ratio (coefficient ratio)
Step 10:
How do I set it up?
Answer: units first, set up the units that you need to cancel out (in red)
| 18 H2O | 1 mol | O2 | L O2 | |
| 18 | H2O |
Step 11:
What is the next step?
Answer: Fill in the numbers and cross out units
| 18 H2O | 1 mol | 17 O2 | L O2 | |
| 18 | 12 H2O |
Step 12:
Simplify
| 18 | 1 mol | 17 O2 | L O2 | |
| 18 | 12 |
Step 13:
What is the next conversion?
Answer: STP ratio (liters to mole ratio)
Step 14:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 18 | 1 mol | 17 O2 | L = | L O2 |
| 18 | 12 | mol |
Step 15:
What is the next step?
Answer: Fill in the numbers and cross out units
| 18 | 1 mol | 17 O2 | 22.4 L = | L O2 |
| 18 | 12 | 1 mol |
Step 16:
Simplify
| 18 | 1 | 17 O2 | 22.4 L = | L O2 |
| 18 | 12 | 1 |
Step 17:
How do I know I am done with conversions?
Answer: The only units left are the units that match the answer. In this case L and O2
| 18 | 1 mol | 17 O2 | 22.4 L = | L O2 |
| 18 | 12 | 1 |
Step 18:
How do I do the calculations?
Answer: (18 * 17 * 22.4) / (18 * 12) = 31.7
| 18 | 1 mol | 17 O2 | 22.4 L = | 31.7 L O2 |
| 18 | 12 | 1 |
COMPLETE ANSWER: 31.7 L O2
PRACTICE PROBLEMS: Solve these gas stoichiometry problems. Don’t forget to use the periodic table and the conversion map when you need it.
At STP 7 L of O2 gas can make how many moles of MgO?
2 Mg(s) + O2(g) —> 2 MgO(s)
Answer: 0.625 mol MgO
How many Liters of Br2 gas at STP can be made from a sample of 26 grams of AlBr3?
2 AlBr3(s) —> 2 Al(s) + 3 Br2(g)
Answer: 3.27 L Br2
At STP 0.3 L of CO2 requires how many molecules of H2O to completely react?
CH4(g) + 2 O2(g) ——> CO2(g) + 2 H2O(g)
Answer: 1.61 * 1022 molecules H2O
How many Liters of H2 gas at STP are required to completely react with a sample of 4.5 Liters of O2?
6 C(s) + 6 H2(g) + 3 O2(g) ——> C6H12O6(s)
Answer: 9 L O2
Chem – STP (Standard Temperature and Pressure)
What is STP?
STP stands for the Standard Temperature and Pressure. It should be more correctly named the standard temperature and pressure of human inhabitants on earth. That is to say it is approximately the average of the pressure and temperature that most humans live at during most of their lives. The standard temperature is 0 C or 273 K and the standard pressure is 1 atm or 1.013 * 105 Pa. To put that into perspective, the standard pressure is the pressure at approximately sea level. Since about 80% of people live within about 100km (60 miles) of a coastline, that actually makes a lot of sense. But what about the temperature, doesn’t that seem a little low? Well if you think about all the places people live on the earth, the temperatures actually range from about 40 C (a hot dessert) to about -30 C (in the tundra). So to say that 0 C is average is not a stretch. It also makes it easy to remember with only one digit. Although most humans prefer a temperature of about 25 C. All that STP is meant to do is give a quick reference for a person who is trying to calculate a gas under the average conditions where that container might be needed. In other words, if you were to design a container to hold gas that could go anywhere on the surface of the earth at a moments notice these are pretty good guides to the conditions you want to assume. How can STP be used in a problem? Most commonly it is used in an ideal gas law problem (PV = nRT) or in a dynamic gas law problems like (P1V1 / T1 = P2V2 / T2). I will give an example of each below and then a demonstrated example.
Examples: Solve for the following STP gas law problems. Remember STP is 0 C and 1 atm. The gas constant R is 0.08206.
What is the volume of a container of 1.4 mols of H2 gas at STP?
Answer: 31 L….the type of gas H2 has nothing to do with this problem.
If a gas at 5 atm 300K and 0.13L is changed to STP conditions what is the new volume?
Answer: 0.59 L….use combined gas law (P1V1 / T1 = P2V2 / T2).
VIDEO STP Gas Law Demonstrated Example 1 (Ideal Gas Law): How many moles is a gas at 4.9L under STP(1 atm and 273 K) conditions? The gas constant R is 0.08206 if you are in atm, L, mol, K.
Step 1:
What information are we given?
Answer:
V = 4.9 L
P = 1 atm
T = 0 C
R = 0.08206
Step 2:
What conversions are needed?
Answer:
V = 4.9 L
P = 1 atm
T = 0 C ——> 273 K
R = 0.08206
Step 3:
What does the question ask for?
Answer: n = ?
Step 4:
How do we set up the problem?
Answer: Start with the equation
| PV = | nRT |
| 1 | 1 |
Step 5:
What can we fill in for the equation?
Answer: The information we are given (red).
| 1 atm * 4.9 L = | n * 0.08206 * 273 K |
| 1 | 1 |
Step 6:
How we do rearrange the equation?
Answer: Divide both sides by the gas constant and the temperature (red).
| 1 atm * 4.9 L = | n * 0.08206 * 273 K |
| 0.08206 * 273 K | 0.08206 * 273 K |
Step 7:
Cross out the 0.08206 and the 273 K on the right side.
| 1 atm * 4.9 L = | n * 0.08206 * 273 K |
| 0.08206 * 273 K | 0.08206 * 273 K |
Step 8:
Simplify
| 1 atm * 4.9 L = | n |
| 0.08206 * 273 K |
Step 9:
How do I do the calculations?
Answer: (1 * 4.9) / (0.08206 * 273) = 0.219
| 1 atm * 4.9 L = | 0.219 moles |
| 0.08206 * 273 K |
Step 10:
What is the complete answer?
COMPLETE ANSWER: 0.219 mols
VIDEO STP Gas Law Demonstrated Example 2 (Combined Gas Law): At STP (1 atm and 273 K) the volume of a gas is 6.3 L. What is the pressure of the same gas at 7.8 L and 340 K?
Step 1:
What information are we given and what do they ask for?
Answer: Group them into two categories (those that came first in the sentence versus those that came next in the sentence).
Category 1
P1 = 1 atm
V1 = 6.3 L
T1 = 273 K
Category 2
P2 = ?
V2 = 7.8 L
T2 = 340 K
Step 2:
What gas law equation does this fall into?
Answer: Set up the dynamic gas law equation to start.
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Step 3:
Which terms or variables are missing from the information of the question?
Answer: n, so cross that off of the equation
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Step 4:
Simplify
| P1V1 = | P2V2 |
| T1 | T2 |
Step 5:
What can we fill in for the equation?
Answer: The information we are given (red).
| 1 * 6.3 = | P2 * 7.8 |
| 273 | 340 |
Step 6:
How do we rearrange the equation?
Answer: Multiply both sides by 340 and divide both sides by 7.8 (red).
| 1 * 6.3 * 340 = | P2 * 7.8 * 340 |
| 273 * 7.8 | 340 * 7.8 |
Step 7:
Cross out the 340 and the 7.8 on the right side
| 1 * 6.3 * 340 = | P2 * 7.8 * 340 |
| 273 * 7.8 | 340 * 7.8 |
Step 8:
Simplify
| 1 * 6.3 * 340 = | P2 |
| 273 * 7.8 |
Step 9:
How do I do the calculations?
Answer: (1 * 6.3 * 340) / (273 * 7.8) = 1
| 1 * 6.3 * 340 = | 1 atm |
| 273 * 7.8 |
Step 10:
What is the complete answer?
COMPLETE ANSWER: 1 atm
PRACTICE PROBLEMS: Solve these STP gas law problems below. Remember STP means (1 atm and 273 K) and the gas constant R is 0.08206 if you are in atm, L, mol, K.
How many liters is a gas with 43 mol under STP conditions?
Answer: 964 L
If a gas is 9 L under conditions of STP. How many moles of gas do you have?
Answer: 24.9 mol
If you take a gas from STP conditions and then increase the pressure to 7.5 atm. What will be the new temperature assuming the moles stay constant?
Answer: 2048 K
At STP the volume of a gas is 2.1 L. What is the volume of the same gas at 3.4 atm and 700 K?
Answer: 1.58 L
Chem – Dynamic Gas Laws
What are Boyle’s Law, Charles’ Law, Gay-Lusac’s Law, Combined Gas Law…?
I call these the dynamic gas laws because dynamic means changing and some kind of change is exactly what these gas laws are describing. These laws are meant to be useful when you have some kind of container of gas at certain conditions (of pressure, volume, moles, and temperature) and then several minutes or seconds later you change some of the conditions (of pressure, volume, moles, and temperature). These laws allow you to predict the future of what will happen to a gas if started at one set of conditions and then move to another set of conditions. This is key in fields like astrophysics, resource storage, resource mining, space exploration, and undersea exploration. These dynamic gas laws will NEVER use the gas constant of 0.08206.
How did the dynamic gas laws come about? Well, I could go into an in depth history of how all these people that lived long ago whom you have probably never heard about before worked painstakingly hard to come up with these laws that we benefit from every day. However, that will bore you and drag you away from the point I am trying to make. The point is that you only need simple mathematics manipulations to uncover the dynamic gas laws from the ideal gas law (PV = nRT).
If you take two ideal gas law equations and put them side by side of each other they look like what I have below.
| P1V1 = n1R1T1 | P2V2 = n2R2T2 |
| 1 | 1 |
Because the gas constant R is always they same number we can take it away from both sides.
| P1V1 = n1T1 | P2V2 = n2T2 |
| 1 | 1 |
Now we sweep all the variables to one side of the equals sign on each the right and left side.
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Last we set them equal to each other.
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Another way to write that equation would be.
(P1V1 / n1T1) = (P2V2 / n2T2)
This equation in either from will help you solve any dynamic gas law problem like Boyle’s Law, Charles’ Law, Gay-Lusac’s Law, Combined Gas Law. I call the equation above the dynamic gas law equation. No one else calls it that so don’t type it in google and look for it. Keep in mind answering dynamic gas law questions is all about your problems solving skills. Make sure you know how to organize the information in the word problem well before you start in this section. Go back to other sections like……. if you are having problems here. With the following demonstrated examples I will prove my point on how to use math and problems solving skills together to achieve and answer.
VIDEO Dynamic Gas Law Demonstrated Example 1 (Boyle’s Law): If a gas at 2.3 atm and 6.1L becomes 4.5L, what will be the new pressure?
Step 1:
What information are we given and what do they ask for?
Answer: Group them into two categories (those that came first in the sentence versus those that came next in the sentence).
Category 1
P1 = 2.3 atm
V1 = 6.1 L
Category 2
P2 = ?
V2 = 4.5 L
Step 2:
What gas law equation does this fall into?
Answer: Set up the dynamic gas law equation to start.
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Step 3:
Which terms or variables are missing from the information of the question?
Answer: n and T, so cross them off of the equation
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Step 4:
Simplify
| P1V1 = | P2V2 |
| 1 | 1 |
Step 5:
What can we fill in for the equation?
Answer: The information we are given (red).
| 2.3 atm * 6.1 L = | P2 * 4.5 L |
| 1 | 1 |
Step 6:
How do we rearrange the equation?
Answer: Divide both sides by 4.5 L (red).
| 2.3 atm * 6.1 L = | P2 * 4.5 L |
| 4.5 L | 4.5 L |
Step 7:
Cross off 4.5 L from right side.
| 2.3 atm * 6.1 L = | P2 * 4.5 L |
| 4.5 L | 4.5 L |
Step 8:
Simplify
| 2.3 atm * 6.1 L = | P2 |
| 4.5 L |
Step 9:
How do I do the calculations?
Answer: (2.3 * 6.1) / 4.5 = 3.12
| 2.3 atm * 6.1 L = | 3.12 atm |
| 4.5 L |
Step 10:
What is the complete answer?
COMPLETE ANSWER: 3.12 atm
VIDEO Dynamic Gas Law Demonstrated Example 2 (Charles’ Law): What is the volume of a gas at 30 C and constant moles if its volume at 15 C is 920mL?
Step 1:
What information are we given and what do they ask for?
Answer: Group them into two categories (those that came first in the sentence versus those that came next in the sentence). Since moles is constant you don’t need to include n in your information.
Category 1
V1 = ?
T1 = 30 C
Category 2
V2 = 920 mL
T2 = 15 C
Step 2:
What conversions are needed?
Answer:
Category 1
V1 = ?
T1 = 30 C —> 303 K
Category 2
V2 = 920 mL
T2 = 15 C —> 288 K
Step 3:
What gas law equation does this fall into?
Answer: Set up the dynamic gas law equation to start.
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Step 4:
Which terms or variables are missing from the information of the question?
Answer: P and n, so cross them off of the equation
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Step 5:
Simplify
| V1 = | V2 |
| T1 | T2 |
Step 6:
What can we fill in for the equation?
Answer: The information we are given (red).
| V1 = | 920 mL |
| 303 K | 288 K |
Step 7:
How do we rearrange the equation?
Answer: Multiply both sides by 303 K (red).
| 303 K * V1 = | 920 mL * 303 K |
| 303 K | 288 K |
Step 8:
Cross out the 303 K on the left side
| 303 K * V1 = | 920 mL * 303 K |
| 303 K | 288 K |
Step 9:
Simplify
| V1 = | 920 mL * 303 K |
| 288 K |
Step 10:
How do I do the calculations?
Answer: (920 * 303) / 288 = 968
| 968 mL = | 920 mL * 303 K |
| 288 K |
Step 11:
What is the complete answer?
COMPLETE ANSWER: 968 mL
VIDEO Dynamic Gas Law Demonstrated Example 3 (Combined Gas Law): What is the temperature of a gas at 500 mm Hg and 750 mL if it was at 55 C, 0.8L, and 1.2 atm?
Step 1:
What information are we given and what do they ask for?
Answer: Group them into two categories (those that came first in the sentence versus those that came next in the sentence).
Category 1
P1 = 500 mm Hg
V1 = 750 mL
T1 = ?
Category 2
P2 = 1.2 atm
V2 = 0.8 L
T2 = 55 C
Step 2:
What conversions are needed?
Answer:
Category 1
P1 = 500 mm Hg
V1 = 750 mL
T1 = ?
Category 2
P2 = 1.2 atm —> 912 mm Hg
V2 = 0.8 L —> 800 mL
T2 = 55 C —> 328 K
Step 3:
What gas law equation does this fall into?
Answer: Set up the dynamic gas law equation to start.
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Step 4:
Which terms or variables are missing from the information of the question?
Answer: n, so cross that off of the equation
| P1V1 = | P2V2 |
| n1T1 | n2T2 |
Step 5:
Simplify
| P1V1 = | P2V2 |
| T1 | T2 |
Step 6:
What can we fill in for the equation?
Answer: The information we are given (red).
| 500 * 750 = | 912 * 800 |
| T1 | 328 |
Step 7:
How do we rearrange the equation?
Answer: Multiply both sides by T1 and 328 (red).
| 500 * 750 * 328 * T1 = | 912 * 800 * 328 * T1 |
| T1 | 328 |
Step 8:
Simplify
| 500 * 750 * 328 = | 912 * 800 * T1 |
| 1 | 1 |
Step 9:
How do we further rearrange the equation?
Answer: Divide both sides by 912 and 800 (red).
| 500 * 750 * 328 = | 912 * 800 * T1 |
| 912 * 800 | 912 * 800 |
Step 10:
Cross out the 912 and 800 on the right side.
| 500 * 750 * 328 = | 912 * 800 * T1 |
| 912 * 800 | 912 * 800 |
Step 11:
Simplify
| 500 * 750 * 328 = | T1 |
| 912 * 800 |
Step 12:
How do I do the calculations?
Answer: (500 * 750 * 328) / (912 * 800) = 168.6
| 500 * 750 * 328 = | 168.6 K |
| 912 * 800 |
Step 13:
What is the complete answer?
COMPLETE ANSWER: 169 K
PRACTICE PROBLEMS: Solve the dynamic gas law equations below. Remember to always convert Celsius temperature to Kelvin.
If a gas at 3.2 atm and 9.4L is allowed to expand to 17.8L, what will be the new pressure?
Answer: 1.69 atm
What is the volume of a gas at 960 mm Hg if it was 210 mm Hg and 840 mL?
Answer: 184 mL
What is the volume of a gas at 65 C and constant moles if its volume at 25 C is 76 mL?
Answer: 86 mL
If a gas at 21 C is 31 L and we change the volume to 65L. What will be the new temperature in Celcius?
Answer: 343 C
If a gas at 3.6 atm and 400 K is compressed to 2.4 atm what will its temperature be?
Answer: 267 K
If 58 mol of a gas takes up 90L. How many moles would the same gas take up if it is 56L?
Answer: 36 mol
What is the temperature of a gas at 420 mm Hg and 890 mL if it was at 108 C, 2.3 L, and 2.5 atm?
Answer: 32.6 K
Chem – The Ideal Gas Law
What is the ideal gas law?
The ideal gas law is an equation. What the equation describes is how you can manipulate different factors to control how a gas behaves. There are 4 factors in the ideal gas law; pressure, volume, moles, and temperature. I have already explained pressure in the previous section so here I will give a brief description of volume, moles, and temperature. Volume is how much space something takes up. Moles are how many or how much stuff you have (gas molecules or particles in this case). Temperature is how fast the molecules or particles of gas are moving. The ideal gas law equation is below (it is very important equation throughout the rest of your chemistry learning):
PV = nRT
The letter P represents Pressure, the letter V represents Volume, the letter n represents Moles, the letter R represents a constant (a certain number to make the equation work together), the letter T represents temperature. The most common units you will see for the ideal gas law are pressure in atmospheres (atm), volume in Liters (L), moles in moles (mol), and temperature in (K). If the equation has all the units stated in the previous sentence then the constant R is 0.08206. It also has the units of (atm * L / mol * K). However, if the units of the equation change the constant number will also change. They usually call R the gas constant in chemistry books and classes.
The most important thing to know about all gas law equations is that they require you to use the Kelvin temperature scale, which is why I taught you how to convert to the Kelvin temperature scale before I started this section. All other units of pressure, volume, and moles can possibly have different units and the gas law equation will still work (although other units tend to make it more difficult because you have to recalculate what the gas constant R will be). However, YOU CAN NEVER USE ANY OTHER UNITS BESIDES KELVIN FOR THE TEMPERATURE SCALE. Another pain in the ideal gas law problems is that most books and some teachers do not tend to do a good job of telling you that YOU SHOULD ALWAYS HAVE ACCESS TO THE GAS CONSTANT R (0.08206) whenever you are doing homework problems or test. I will now go on to teach you how to solve equations using the ideal gas law. In my demonstrations and example I will not emphasize the units of the gas constant R in the equations because it is too long and complex for this text format. However, if you are more interested in the units of the gas constant then you can click on this link. First let us look at some examples to get an idea of what we will be asked of us.
Examples: Solve for the following ideal gas law problems.
If the volume of a gas container is 3L and the amount of moles is 2.2 moles and the temperature is 273K. What is the Pressure in atmospheres given that the gas constant ( R ) is 0.08206?
Answer: 16.4 atm
A container of gas is 530ml at 600 torr, and 23 C. If the gas constant is 0.08206 how many moles are in the container?
Answer: 0.0172 mol….(don’t forget to convert all the units before you use the ideal gas law)
VIDEO Ideal Gas Law Demonstrated Example 1: If the pressure of a gas is 1.5 atm at 13 mol and 300 K with as gas constant of 0.08206. Then what is the volume?
Step 1:
What information are we given?
Answer:
P = 1.5atm
n = 13 mol
R = 0.08206
T = 300 K
Step 2:
What does the question ask for?
Answer: V = ?
Step 3:
How do we set up the problem?
Answer: Start with the equation
| PV = | nRT |
| 1 | 1 |
Step 4:
What can we fill in for the equation?
Answer: The information we are given (red).
| 1.5 atm * V = | 13 mol * 0.08206 * 300 K |
| 1 | 1 |
Step 5:
How do we rearrange the equation?
Answer: Divide both sides by pressure (red)
| 1.5 atm * V = | 13 mol * 0.08206 * 300 K |
| 1.5 atm | 1.5 atm |
Step 6:
Cross off 1.5 atm from the left side.
| 1.5 atm * V = | 13 mol * 0.08206 * 300 K |
| 1.5 atm | 1.5 atm |
Step 7:
Simplify
| V = | 13 mol * 0.08206 * 300 K |
| 1.5 atm |
Step 8:
How do I do the calculations?
Answer: (13 * 0.08206 * 300) / 1.5 = 213
| 213 L = | 13 mol * 0.08206 * 300 K |
| 1.5 atm |
Step 9:
What is the complete answer?
COMPLETE ANSWER: 213 L
VIDEO Ideal Gas Law Demonstrated Example 2: If a gas is 250 mL and 600 mmHg at 230 C, how many moles will that gas be if the gas constant is 0.08206?
Step 1:
What information are we given?
Answer:
V = 250 mL
P = 600 mmHg
T = 230 C
R = 0.08206
Step 2:
What conversions are needed?
Answer:
V = 250 mL ——> 0.25 L
P = 600 mmHg ——> 0.789 atm
T = 230 C ——> 503 K
R = 0.08206
Step 3:
What does the question ask for?
Answer: n = ?
Step 4:
How do we set up the problem?
Answer: Start with the equation
| PV = | nRT |
| 1 | 1 |
Step 5:
What can we fill in for the equation?
Answer: The information we are given (red).
| 0.789 atm * 0.25 L = | (n) * 0.08206 * 503 K |
| 1 | 1 |
Step 6:
How do we rearrange the equation?
Answer: Divide both sides by the gas constant and the temperature (red).
| 0.789 atm * 0.25 L = | (n) * 0.08206 * 503 K |
| 0.08206 * 503 K | 0.08206 * 503 K |
Step 7:
Cross off 0.08206 and 503 K from the right side
| 0.789 atm * 0.25 L = | (n) * 0.08206 * 503 K |
| 0.08206 * 503 K | 0.08206 * 503 K |
Step 8:
Simplify
| 0.789 atm * 0.25 L = | (n) |
| 0.08206 * 503 K |
Step 9:
How do I do the calculations?
Answer: (0.789 * 0.25) / (0.08206 * 503) = 0.004779
| 0.789 atm * 0.25 L = | 0.004779 mol |
| 0.08206 * 503 K |
Step 10:
What is the complete answer?
COMPLETE ANSWER: 0.00478 mol…or…4.78 * 10-3 mol
PRACTICE PROBLEMS: Solve the Ideal gas law equations below. Keep in mind the gas constant is always 0.08206 if you are in atm, L, mol, and K and that you can use that number with any of these problems.
If the pressure of a gas is 4 atm at 3 mol and 250 K, then what is the volume?
Answer: 15.4 L
If the volume of a gas is 5 L at 42 C and 2.5 mol, then what is the pressure?
Answer: 12.9 atm….(convert Celsius to Kelvin)
How many moles are in a container of gas that is 940 torr at 25 C and 0.2 L?
Answer: 0.01014 mol…..(convert torr and Celsius)
A gas that is 1.05 * 106 Pa, 710 mL, and 9 mol is at what temperature in Celcius?
Answer: -263 C….(Convert everything before you put it into the equations then convert the temperature after you are done with the equation).
Chem – Temperature Conversions
How do temperature conversions work?
If you want to know more about temperature go back to the temperature section in the states of matter lesson. What you need to learn from this section is how to convert between the temperature scales (units to measure temperature). Because temperature devices were so difficult to make until about 1900, different people came up with different temperature scales. As a result, we are left with some temperature scales that are quite useless. The Fahrenheit temperature scale is one of those relics that is left over. The daily or morning news in America gives predicted temperatures in the Fahrenheit scale. However, the Celsius scale is a far better temperature measurement system for us to use on earth. If you want to know how to convert between Fahrenheit and Celsius scale go to this link. Most students don’t need to know how to convert between Fahrenheit and Celsius so I will not teach it on this page. The Celsius scale was set up to revolve around the temperature that water will freeze at on earth if the Celsius measurement is zero. This is significant to all life on Earth, since all life on earth needs water to survive. However, after you leave our tiny little planet of Earth the Celsius scale loses it meaning. That is why the Kelvin scale was created. The Kelvin scale works everywhere in the universe, which is the best kind of scale to use for any mathematical calculations you have to do (like chemistry formulas). The reason why it works anywhere is because the Kelvin scale is a true measurement of how molecules or particles move. At zero degrees Kelvin all molecule or particle movement stops. This is why we will eventually use the Kelvin scale for nearly all of our chemistry calculations. Now lets try looking at the formula for converting between the Celsius temperature scale and the Kelvin temperature scale.
Celsius to Kelvin:
K = C + 273
The letter K represents the temperature in Kelvin and the letter C represents the temperature in Celsius.
Examples: Convert the following temperature units.
What is the Kelvin measurement of 150 C?
Answer: 423 K
What is the Celsius measurement of 240K?
Answer: -33 C
VIDEO Temperature Conversions Demonstrated Example 1: If the temperature is 25 C what is the Kelvin temperature?
Step 1:
What information are we given?
Answer: 25 C
Step 2:
What units does the question ask for?
Answer: Kelvin
Step 3:
How do we set up the problem?
Answer: Start with the equation
| K = | C + 273 |
Step 4:
What can we fill in for the equation?
Answer: The information we are given (red).
| K = | 25 + 273 |
Step 5:
How do I do the calculations?
25 + 273 = 298
Step 6:
What is the complete answer?
COMPLETE ANSWER: 298 K
VIDEO Temperature Conversions Demonstrated Example 2: If the temperature is 800 K what is the Celsius temperature?
Step 1:
What information are we given?
Answer: 800 K
Step 2:
What units does the question ask for?
Answer: Celsius
Step 3:
How do we set up the problem?
Answer: Start with the equation
| K = | C + 273 |
Step 4:
What can we fill in for the equation?
Answer: The information we are given (red).
| 800 = | C + 273 |
Step 5:
How do we rearrange the equation?
Answer: Subtract 273 from both sides
| 800 -273 = | C + 273 -273 |
Step 6:
Simplify by canceling the positive and negative 273 on the right side.
| 800 -273= | C |
Step 7:
How do I do the calculations?
Answer: 800 – 273 = 527
Step 8:
What is the complete answer?
COMPLETE ANSWER: 527 C
PRACTICE PROBLEMS: Convert between Kelvin and Celsius in the questions below.
What is the Kelvin temperature if it is 312 C?
Answer: 585 K
What is the Celsius temperature if it is 218 K?
Answer: -55 C
What is the Kelvin temperature if it is 40 C?
Answer: 313 K
What is the Celsius temperature if it is 382 K?
Answer: 109 C
Chem – Pressure Conversions
How do you do conversions between pressure units?
Fundamentally, the pressure of a gas is how many times and with how much force are the molecules of air striking the container. It is like a massage. The person giving you a massage can strike your skin in many different ways. They can strike hard and often which would be high pressure or they can strike soft and infrequent which would be low pressure. The same is true with the molecules of air in a container like a balloon. The molecules can create a high pressure by striking hard and often or a low pressure by striking soft and infrequent. How we influence the molecules to create a high pressure or a low pressure? We can change several factors like the number of molecules we have, the volume of the container, and the temperature. All of the factors that influence pressure I will discuss later.
If you want additional explanation and descriptions of pressure then go to the pressure section the states of matter lesson.
What we need to know right now is how pressure is measured. Specifically, the units of pressure. The units of pressure include Pascal (Pa), atmospheres (atm), torr, and mm Hg. Torr and mm Hg are really the same thing where as Pascals and atmospheres are different from all others. A Pascal is the standard unit of measurement for the metric scale (the SI unit), however, atmospheres tend to be the most popular unit to use for pressure in a chemistry class. What are the conversions between the units of pressure?
1 atm = 1.013 * 105 Pa = 760 torr = 760 mm Hg
Each of these can be written as ratios or proportions like below:
Atm:
| 1.013 * 105 Pa | 760 torr | 760 mm Hg | ||
| 1 atm | 1 atm | 1 atm |
Pa:
| 1 atm | 760 torr | 760 mm Hg | ||
| 1.013 * 105 Pa | 1.013 * 105 Pa | 1.013 * 105 Pa |
Torr:
| 1.013 * 105 Pa | 1 atm | 760 mm Hg | ||
| 760 torr | 760 torr | 760 torr |
mm Hg:
| 1.013 * 105 Pa | 1 atm | 760 torr | ||
| 760 mm Hg | 760 mm Hg | 760 mm Hg |
The ratios above are much like the ones we have used in other sections like unit conversions. If you have a hard time with the examples go back to the lesson just mentioned.
Examples: Convert the following pressure units.
How many mm Hg is a pressure of 2.3 atm?
Answer: 1748 mm Hg
If the pressure is 89 torr. How many pascals is that?
Answer: 11862 Pa
VIDEO Pressure Conversions Demonstrated Example 1: How many atmospheres is 450 torr?
Step 1:
What information are we given?
Answer: 450 torr
Step 2:
What units does the question ask for?
Answer: atm
Step 3:
How do we set up the problem?
Answer:
| 450 torr | atm | |
| 1 |
Step 4:
What is the first conversion?
Answer: 1 atm = 760 torr
Step 5:
How do we set it up?
Answer: units first, set up the units that need to cancel out (in red)
| 450 torr | atm = | atm |
| torr |
Step 6:
What comes next?
Answer: fill in the numbers and cross out units
| 450 torr | 1 atm = | atm |
| 760 torr |
Step 7:
Simplify by removing all crossed out units. The units in red are the ones that are left and match the answer units also in red.
| 450 | 1 atm = | atm |
| 760 |
Step 8:
How do I do the calculations?
Answer: 450 / 760 = 0.59
| 450 | 1 atm = | 0.59 atm |
| 760 |
Step 9:
What is the complete answer?
COMPLETE ANSWER: 0.59 atm
VIDEO Pressure Conversions Demonstrated Example 2: If the pressure is 2.4 * 104 Pa, how many mm Hg is that?
Step 1:
What information are we given?
Answer: 2.4 * 104 Pa
Step 2:
What units does the question ask for?
Answer: mm Hg
Step 3:
How do we set up the problem?
Answer:
| 2.4 * 104 Pa | mm Hg | |
| 1 |
Step 4:
What is the first conversion?
Answer: 1.013 * 105 Pa = 760 mm Hg
Step 5:
How do we set it up?
Answer: units first, set up the units that need to cancel out (in red)
| 2.4 * 104 Pa | mm Hg = | mm Hg |
| Pa |
Step 6:
What comes next?
Answer: fill in the numbers and cross out units
| 2.4 * 104 Pa | 760 mm Hg = | mm Hg |
| 1.013 * 105 Pa |
Step 7:
Simplify by removing all crossed out units. The units in red are the ones that are left and match the answer units also in red.
| 2.4 * 104 | 760 mm Hg = | mm Hg |
| 1.013 * 105 |
Step 8:
How do I do the calculations?
Answer: (2.4 * 104 * 760) / (1.013 * 105) = 180
| 2.4 * 104 | 760 mm Hg = | 180 mm Hg |
| 1.013 * 105 |
Step 9:
What is the complete answer?
COMPLETE ANSWER: 180 mm Hg
PRACTICE PROBLEMS: Give the requested units of pressure for the questions below.
How many atmospheres is 360 torr?
Answer: 0.473atm
How many mm Hg is 2.3atm?
Answer: 1748 mm Hg
5.9atm is how many Pa?
Answer: 5.98 * 105 Pa
824 mm Hg is how many atmospheres?
Answer: 1.08atm
Chem – Kinetic Molecular Theory
What is the Kinetic Molecular Theory?
This is a review of the subject but for a more complete explanation also check out the molecules and states of matter section in the states of matter lesson. To understand gasses, we must first understand what is called the kinetic molecular theory. What is the kinetic molecular theory? Let us break down the phrase. KIN means movement, MOLECUL means molecule. It is a theory of how molecules move. This happens for all molecules, but is most easily understood in a gas. Imagine if you and 2000 of your friends each had a soccer ball on a smooth surface like a small iced over lake. If all of you stood around the edge of the lake in a circle and kicked your soccer ball into the middle of the lake and then kept kicking every soccer ball that came your way back into the middle of the lake. That would be how the molecules of air are acting in the air around you. They would move across the lake and bump into each other and bounce or travel toward the outer edge. The only difference between the lake example and air molecules is that in the lake example we are demonstrating what is looks like in 2 dimensions. In reality molecules in the air bounce around in 3 dimensions. I have found the best way to imagine the reality of how air moves is think about it inside a container like a balloon. All the air molecules are bouncing around inside balloon like little soccer balls. They hit each other they hit the inside walls the balloon container and they just keep bouncing around. From the visualization of the kinetic molecular theory, we can begin to ask how is the way that gases behave reflected in gas law formulas?
Chem – LESSON 13: Gas Laws
What is the lesson about?
This lesson is about how gases behave. It allows you to define them in terms of several characteristics. It also teaches you about more simple definitions like pressure and temperature and more complicated situations like how gas can change under different conditions like temperature.
Why is it critical to understand?
Learning how gases work prepares you with information for later lessons like reaction rate, equilibrium, and electrochemistry. It also has many real world applications such as telling you when gas build up can become dangerous, how explosions work, what causes a car engine to move, and why balloons with rise and fall.
What should you know before attempting this lesson?
If you have trouble in this lesson go back to sections on Equations, Solving for an Unknown, Unit Conversions, Calculating the Molar Mass of Compounds, Molecules and States of Matter, States of Matter in a Chemical Equation, Balancing Chemical Equations Part 1, Grams to Moles Conversions and Combining Stoichiometry and Molar Mass.
New Learning Sections:
—> Dynamic Gas Laws (Boyle’s, Charles’, Combined)
—> STP (Standard Temperature and Pressure)
—> Effusion
Reference Pages:
—> Gas Law Equations, Conversions, and Constants Sheet
Worksheets:
Chem – Percent Yield
What is percent yield?
The percent yield section is very simple mathematically. However, the components of the math equation for this section are often not clearly labeled. On top of that, percent yield will quite frequently be mixed in with other concepts like stoichiometry. In light of this, I will try to show a clear definition of the percent yield equation and give examples that are mixed with other concepts. The equation is below.
| Percent Yield = | Actual | * 100 |
| Theoretical |
The hardest part about this equation is to decide what number is your actual and what number is your theoretical. The best guide I have found in chemistry is that the theoretical number almost always comes from a conversion calculation. The actual number usually is accompanied by some kind of phrase like “at the end of the reaction” or “when the reaction is complete”. The theoretical is what you were supposed to get if everything went perfect in the process. However, like life nothing ever goes perfect in chemistry. The actual is the amount you do get when you take into account any mistakes that were made. The percent yield has no units. Which means the actual and theoretical yield have to have the same units when you divide them.
Examples: Give the part of the percent yield calculation missing.
If your actual yield is 17g and your theoretical yield is 43g then what is your percent yield?
Answer: 39.5 %
From the reaction you were supposed to get 21g of CO2 but instead you got 15g of CO2. What was your percent yield?
Answer: 71.4%
If your percent yield of O2 from the reaction below was 86% then what is your actual yield if you started with 63g of H2O?
2 H2O(l) —> 2 H2(g) + O2(g)
Answer: 49.9 g O2 (hint you have to convert the 63g of H2O to get the theoretical)
VIDEO Percent Yield Demonstrated Example 1: Your calculations indicated that you would produce 3.7g of LiOH but instead you produced 5.2g of LiOH. What is your percent yield?
Step 1:
What information does the question supply us with?
Answer:
Actual = 5.2 g LiOH
Theoretical = 3.7 g LiOH
Step 2:
What section of the formula does the question ask?
Answer: percent yield = ?
Step 3:
What is the formula?
| Percent Yield = | Actual | * 100 |
| Theoretical |
Step 4:
Fill in the formula with the information above
| Percent Yield = | 5.2 g LiOH | * 100 |
| 3.7 g LiOH |
Step 5:
How do you do the calculations?
Answer: (5.2 * 100) / (3.7) = 140
| 140 % = | 5.2 g LiOH | * 100 |
| 3.7 g LiOH |
Step 6:
COMPLETE ANSWER: Percent yield = 140 %
VIDEO Percent Yield Demonstrated Example 2: If you had a percent yield of 93% and at the end of the chemical reaction found you weighed out 8.4g. What was your theoretical yield?
Step 1:
What information does the question supply us with?
Answer:
percent yield = 93%
Actual = 8.4 g
Step 2:
What section of the formula does the question ask?
Answer: theoretical yield = ?
Step 3:
What is the formula?
| Percent Yield = | Actual | * 100 |
| Theoretical |
Step 4:
Fill in the formula with the information above
| 93 = | 8.4 g | * 100 |
| Theoretical |
Step 5:
How do you solve for volume?
Answer: First multiply both sides by theoretical (red)
| 93 * Theoretical = | 8.4 g | * 100 * Theoretical |
| Theoretical |
Step 6:
Cross out Theoretical on the right side
| 93 * Theoretical = | 8.4 g | * 100 * Theoretical |
| Theoretical |
Step 7: Simplify
| 93 * Theoretical = | 8.4 g | * 100 |
| 1 |
Step 8:
Now divide both sides by 93 (red)
| 93 * Theoretical = | 8.4 g | * 100 |
| 93 | 93 |
Step 9:
Cross out 93 on the left side
| 93 * Theoretical = | 8.4 g | * 100 |
| 93 | 93 |
Step 10: Simplfy
| Theoretical = | 8.4 g | * 100 |
| 93 |
Step 11:
How do I do the calculations?
Answer: (8.4 * 100) / (93) = 7.8
| 7.8 g = | 8.4 g | * 100 |
| 93 |
Step 12:
COMPLETE ANSWER: 7.8 g
VIDEO Percent Yield Demonstrated Example 3: After the reaction you were left with 9g of NH3. What was your percent yield if you started with 4.2g of H2. You will need the periodic table for this problem.
N2(g) + 3 H2(g) —-> 2 NH3(g)
Step 1:
What information does the question supply us with?
Answer:
Actual = 9 g NH3
Theoretical = 4.2 g H2
Step 2:
What section of the formula does the question ask?
Answer: percent yield = ?
Step 3:
Where do we start?
Answer: We first need to start with a conversion because our actual and theoretical units do not match. We need to convert the theoretical from g H2 to g NH3
Step 4:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 3 arrows when we go from Grams of A —> moles of A —> moles of B —> Grams of B. 3 arrows = 3 conversions
Step 5:
How do we set up the conversion?
Answer:
| 4.2 g H2 | g NH3 | |||
| 1 |
Step 6:
What is the first conversion?
Answer: molar mass (grams to mole ratio) of H2 found on the periodic table
Step 7:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 4.2 g H2 | mol | g NH3 | ||
| g |
Step 8:
What is the next step?
Answer: Fill in the numbers and cross out units
| 4.2 g H2 | 1 mol | g NH3 | ||
| 2 g |
Step 9: Simplify
| 4.2 H2 | 1 mol | g NH3 | ||
| 2 |
Step 10:
What is the next conversion?
Answer: mole to mole ratio (coefficient ratio)
Step 11:
How do I set it up?
Answer: units first, set up the units that you need to cancel out (in red)
| 4.2 H2 | 1 mol | NH3 | g NH3 | |
| 2 | H2 |
Step 12:
What is the next step?
Answer: Fill in the numbers and cross out units
| 4.2 H2 | 1 mol | 2 NH3 | g NH3 | |
| 2 | 3 H2 |
Step 13: Simplify
| 4.2 | 1 mol | 2 NH3 | g NH3 | |
| 2 | 3 |
Step 14:
What is the next conversion?
Answer: molar mass (grams to mole ratio) of H2 found on the periodic table
Step 15:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 4.2 | 1 mol | 2 NH3 | g = | g NH3 |
| 2 | 3 | mol |
Step 16:
What is the next step?
Answer: Fill in the numbers and cross out units
| 4.2 | 1 mol | 2 NH3 | 17 g = | g NH3 |
| 2 | 3 | 1 mol |
Step 17: Simplify
| 4.2 | 1 | 2 NH3 | 17 g = | g NH3 |
| 2 | 3 | 1 |
Step 18:
How do I do the calculations?
Answer: (4.2 * 2 * 17) / (2 * 3) = 23.8
| 4.2 | 1 | 2 NH3 | 17 g = | 22.7 g NH3 |
| 2 | 3 | 1 |
Now we can compare the 23.8 g NH3 which is our theoretical value to our actual value of 9 g NH3.
Step 19:
How do we do that?
Answer: With the percent yield formula
| Percent Yield = | Actual | * 100 |
| Theoretical |
Step 20:
Fill in the numbers
| Percent Yield = | 9 g NH3 | * 100 |
| 23.8 g NH3 |
Step 21:
How do I do the calculations?
Answer: (9 * 100) / (23.8) = 37.8
| 37.8 % = | 9 g NH3 | * 100 |
| 23.8 g NH3 |
Step 20:
COMPLETE ANSWER: 37.8 %
PRACTICE PROBLEMS: Solve these percent yield problems. Don’t forget to use the periodic table and the conversion map when you need them.
Your calculations indicated that you would produce 4.8g but instead you produced 3.2g. What is your percent yield?
Answer: 66.7%
If you had a percent yield of 75% and at the end of the chemical reaction found you weighed out 36g. What was your theoretical yield?
Answer: 48 g
After the reaction you were left with 19g of O2. What was your percent yield if you started with 82g of H2SO4.
H2SO4(aq) —–> H2(aq) + S(s) + 2 O2(aq)
Answer: 35.5%
If you had a 48% yield at the end of the chemical reaction and you started with 65g of CO32-. Then what is your actual yield of Fe2(CO3)3.
2 Fe3+(aq) + 3 CO32-(aq) —-> Fe2(CO3)3(s)
Answer: 50.6 g Fe2(CO3)3
Now try the Stoichiometry worksheet.
Chem – Limited Reactant
What is LIMITED REACTANT (REAGENT)?
Many students have tremendous trouble with this section. However, it should not be so if they have studied the previous section of combining stoichiometry and molar mass conversions. The only difference between limited reactant and previous conversion sections are that limited reactant problems have 2 or more starting points. This means that you usually have to do 2 or more conversions. The same conversions as you have done before, using the same conversion map as the last section. Again, we can turn back to the baking analogy for real life example of limited reactant. If we have the same baking equation below as we had before and I tell you that we start with 5 eggs and 7 cups of sugar, how many cookies can we make? Which ingredient runs out first (which reactant is limiting)? Which ingredient will we have extra after the cookies are made (which reactant will be in excess)?
2 eggs + 3 cups of sugar —> 24 cookies
In this case we can make 56 cookies since the 7 cups of sugar will be the first ingredient to run out. That means that we will have some egg left over because we did not use all of them up.
| 5 eggs | 24 cookies | = 60 cookies |
| 2 eggs |
| 7 cups of sugar | 24 cookies | = 56 cookies |
| 3 cups of sugar |
So the limited reactant is the chemical that will be used up first in a reaction. The excess reactant is the one that will have some amount remaining after the reaction is done.
VIDEO Stoichiometry (Limited Reactant) Conversions Demonstrated Example 6: If you have 12 g of N2 and 3g of H2 then what is your limited reactant? How many grams of NH3 can you make? You will need a periodic table to help solve this problem.
N2(g) + 3 H2(g) —-> 2 NH3(g)
Step 1:
What information does the question supply us with?
Answer: 12 g of N2 and 3g of H2
Step 2:
What units does the question ask?
Answer: the limited reactant and g NH3
Step 3:
Where do we start?
Answer: With either of the pieces of information the questions supplies us with. I will start with 12g of N2.
Step 4:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 3 arrows when we go from Grams of A —> moles of A —> moles of B —> Grams of B. 3 arrows = 3 conversions
Step 5:
How do we set up the first set of conversions for this problem?
Answer:
| 12 g N2 | g NH3 | |||
| 1 |
Step 6:
What is the first conversion?
Answer: molar mass (grams to mole ratio) of N2 found on the periodic table
Step 7:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 12 g N2 | mol | g NH3 | ||
| g |
Step 8:
What is the next step?
Answer: Fill in the numbers and cross out units
| 12 g N2 | 1 mol | g NH3 | ||
| 28 g |
Step 9: Simplify
| 12 N2 | 1 mol | g NH3 | ||
| 28 |
Step 10:
What is the next conversion?
Answer: mole to mole ratio (coefficient ratio)
Step 11:
How do I set it up?
Answer: units first, set up the units that you need to cancel out (in red)
| 12 N2 | 1 mol | NH3 | g NH3 | |
| 28 | N2 |
Step 12:
What is the next step?
Answer: Fill in the numbers and cross out units
| 12 N2 | 1 mol | 2 NH3 | g NH3 | |
| 28 | 1 N2 |
Step 13: Simplify
| 12 | 1 mol | 2 NH3 | g NH3 | |
| 28 | 1 |
Step 14:
What is the next conversion?
Answer: molar mass (grams to mole ratio) of NH3 found on the periodic table
Step 15:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 12 | 1 mol | 2 NH3 | g = | g NH3 |
| 28 | 1 | mol |
Step 16:
What is the next step?
Answer: Fill in the numbers and cross out units
| 12 | 1 mol | 2 NH3 | 17 g = | g NH3 |
| 28 | 1 | 1 mol |
Step 17: Simplify
| 12 | 1 | 2 NH3 | 17 g = | g NH3 |
| 28 | 1 | 1 |
Step 18:
How do I know I am done with this conversion?
Answer: The only units left are the units that match the answer. In this case g and NH3
| 12 | 1 | 2 NH3 | 17 g = | g NH3 |
| 28 | 1 | 1 |
Step 19:
How do I do the calculations?
Answer: (12 * 2 * 17) / (28) = 14.6
| 12 | 1 | 2 NH3 | 17 g = | 14.6g NH3 |
| 28 | 1 | 1 |
ARE WE DONE YET? NNNOOOOOO!!!! We have only one answer (14.6 g NH3) of 2 that we need. Now we have to start all over again with the next piece of information 3g of H2 and end our conversion at the same place.
Step 20:
How do we set up the second set of conversions for this problem?
Answer:
| 3 g H2 | g NH3 | |||
| 1 |
Step 21:
What is the first conversion?
Answer: molar mass (grams to mole ratio) of H2 found on the periodic table
Step 22:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 3 g H2 | mol | g NH3 | ||
| g |
Step 23:
What is the next step?
Answer: Fill in the numbers and cross out units
| 3 g H2 | 1 mol | g NH3 | ||
| 2 g |
Step 24: Simplify
| 3 H2 | 1 mol | g NH3 | ||
| 2 |
Step 25:
What is the next conversion?
Answer: mole to mole ratio (coefficient ratio)
Step 26:
How do I set it up?
Answer: units first, set up the units that you need to cancel out (in red)
| 3 H2 | 1 mol | NH3 | g NH3 | |
| 2 | H2 |
Step 27:
What is the next step?
Answer: Fill in the numbers and cross out units
| 3 H2 | 1 mol | 2 NH3 | g NH3 | |
| 2 | 3 H2 |
Step 28: Simplify
| 3 | 1 mol | 2 NH3 | g NH3 | |
| 2 | 3 |
Step 29:
What is the first conversion?
Answer: molar mass (grams to mole ratio) of NH3 found on the periodic table
Step 30:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 3 H2 | 1 mol | 2 NH3 | g = | g NH3 |
| 2 | 3 H2 | mol |
Step 31:
What is the next step?
Answer: Fill in the numbers and cross out units
| 3 | 1 mol | 2 NH3 | 17 g = | g NH3 |
| 2 | 3 | 1 mol |
Step 32: Simplify
| 3 | 1 | 2 NH3 | 17 g = | g NH3 |
| 2 | 3 | 1 |
Step 33:
How do I know I am done with this conversion?
Answer: The only units left are the units that match the answer. In this case g and NH3
| 3 | 1 | 2 NH3 | 17 g = | g NH3 |
| 2 | 3 | 1 |
Step 34:
How do I do the calculations?
Answer: (3 * 2 * 17) / (2 * 3) = 17
| 3 | 1 | 2 NH3 | 17 g = | 17 g NH3 |
| 2 | 3 | 1 |
Step 35:
NOW WE HAVE TWO ANSWERS? SO WHAT DO WE DO WITH THEM? SINCE THEY ARE THE SAME UNITS WE CAN COMPARE THEM. 17 g of NH3 versus 14.6 g NH3. The one that is less is the one we wish to focus on. From the 14.6 g NH3 we tract back to where we calculated that from. It was from the 12 g N2. So this is our limiting reagent. It makes the least amount of product.
Step 36:
COMPLETE ANSWER: Limited reagent is N2 and we can make 14.6 g NH3
VIDEO Stoichiometry (Limited Reactant) Conversions Demonstrated Example 7: If you have 4 g of Ba(OH)2 and 2 g of HCl then what is your excess reactant? How much of the excess reactant will be left over? You will need a periodic table to help solve this problem.
Ba(OH)2(aq) + 2 HCl(aq) ——> 2 H2O(l) + BaCl2(aq)
Step 1:
What information does the question supply us with?
Answer: 4 g of Ba(OH)2 and 2 g of HCl
Step 2:
What units does the question ask?
Answer: the excess reactant and how much is left over after the reaction occurs
Step 3:
Where do we start?
Answer: With excess reactant problems it is harder to figure out where to start. Basically you want to do a comparison between the two reactants so you want to convert one of them into the units of the other. You can start with either of the pieces of information the questions supplies us with. I will start with 4 g of Ba(OH)2 and convert to g HCl.
Step 4:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 3 arrows when we go from Grams of A —> moles of A —> moles of B —> Grams of B. 3 arrows = 3 conversions
Step 5:
How do we set up the first set of conversions for this problem?
Answer:
| 4 g Ba(OH)2 | g HCl | |||
| 1 |
Step 6:
What is the first conversion?
Answer: molar mass (grams to mole ratio) of Ba(OH)2 found on the periodic table
Step 7:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 4 g Ba(OH)2 | mol | g HCl | ||
| g |
Step 8:
What is the next step?
Answer: Fill in the numbers and cross out units
| 4 g Ba(OH)2 | 1 mol | g HCl | ||
| 171 g |
Step 9: Simplify
| 4 Ba(OH)2 | 1 mol | g HCl | ||
| 171 |
Step 10:
What is the next conversion?
Answer: mole to mole ratio (coefficient ratio)
Step 11:
How do I set it up?
Answer: units first, set up the units that you need to cancel out (in red)
| 4 Ba(OH)2 | 1 mol | HCl | g HCl | |
| 171 | Ba(OH)2 |
Step 12:
What is the next step?
Answer: Fill in the numbers and cross out units
| 4 Ba(OH)2 | 1 mol | 2 HCl | g HCl | |
| 171 | 1 Ba(OH)2 |
Step 13: Simplify
| 4 | 1 mol | 2 HCl | g HCl | |
| 171 | 1 |
Step 14:
What is the next conversion?
Answer: molar mass (grams to mole ratio) of Ba(OH)2 found on the periodic table
Step 15:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 4 | 1 mol | 2 HCl | g = | g HCl |
| 171 | 1 | mol |
Step 16:
What is the next step?
Answer: Fill in the numbers and cross out units
| 4 | 1 mol | 2 HCl | 36 g = | g HCl |
| 171 | 1 | 1 mol |
Step 17: Simplify
| 4 | 1 | 2 HCl | 36 g = | g HCl |
| 171 | 1 | 1 |
Step 18:
How do I know I am done with this conversion?
Answer: The only units left are the units that match the answer. In this case g and HCl
| 4 | 1 | 2 HCl | 36 g = | g HCl |
| 171 | 1 | 1 |
Step 19:
How do I do the calculations?
Answer: (4 * 2 * 36) / (171) = 1.68
| 4 | 1 | 2 HCl | 36 g = | 1.68g HCl |
| 171 | 1 | 1 |
Step 20:
We can now compare this conversion answer of 1.68 g HCl to the original piece of information we have of 2 g of HCl. We can only compare these because they have the same units. Since 2 g HCl is higher than the 1.68 g of HCl the HCl is the excess reactant. How much of the excess reactant is left over? For that we can take the difference between the two numbers.
2 g HCl – 1.68 g HCl = 0.32 g HCl
Step 21:
COMPLETE ANSWER: HCl is the excess reactant and 0.32 g of HCl is left after the reaction.
PRACTICE PROBLEMS: Solve these limited reactant stoichiometry problems. Don’t forget to use the periodic table and the conversion map when you need them.
If you have 15g of C4 and 20g of O2 then what is your limited reactant? How many grams of CO2 can you make?
C4(s) + 4 O2(g) —-> 4 CO2(g)
Answer: O2 is the limiting reactant and 27.5 g of CO2 can be made
If you have 7 g of CO32- and 6 g of Fe3+ then what is your limited reactant? How many grams of Fe2(CO3)3 can you make?
2 Fe3+(aq) + 3 CO32-(aq) —-> Fe2(CO3)3(s)
Answer: CO32- is the limiting reactant and 11.36 g of Fe2(CO3)3 can be made
If you have 0.6 g of MgBr2 and 0.9 g of NaI then what is your excess reactant? How much of the excess reactant will be left over?
MgBr2(aq) + 2 NaI(aq) ——> MgI2(aq) + 2 NaBr(aq)
Answer: MgBr2 is your excess reactant and 0.078 g of MgBr2 is left after the reaction
If you have 18 g of C4H10 and 30 g of O2 then what is your excess reactant? How much of the excess reactant will be left over?
2 C4H10 (l) + 13 O2 (g) ——> 8 CO2 (g) + 10 H2O(g)
Answer: C4H10 is the excess reactant and 99g of C4H10 is left after the reaction.
Chem – Combining Stoichiometry and Molar Mass Conversions
COMBINING STOICHIOMETRY AND MOLAR MASS CONVERSIONS (MOLES TO GRAMS CONVERSIONS):
Now that we are more comfortable with stoichiometry, we can combine it with our previous efforts of molar mass conversions in the how to convert between grams and moles section. This means we are going to further complicate our conversion map to include more destinations.
VIDEO Stoichiometry Conversions Demonstrated Example 3: If you have 4 mol of O2 then how many grams of H2 will you need to completely react? You will need a periodic table to help solve this problem.
2 H2(g) + O2(g) —-> 2 H2O(l)
Step 1:
What information does the question supply us with?
Answer: 4 mol O2
Step 2:
What units does the question ask?
Answer: g H2
Step 3:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 2 arrows when we go from Moles of A —> Moles of B —> Grams of B. 2 arrows = 2 conversions
Step 4:
How do we set up the problem?
Answer:
| 4 mol O2 | g H2 | ||
| 1 |
Step 5:
What is the first conversion?
Answer: mole to mole ratio (coefficient ratio)
Step 6:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 4 mol O2 | H2 | g H2 | |
| O2 |
Step 7:
What is the next step?
Answer: Fill in the numbers and cross out units
| 4 mol O2 | 2 H2 | g H2 | |
| 1 O2 |
Step 8: Simplify
| 4 mol | 2 H2 | g H2 | |
| 1 |
Step 9:
What is the next conversion?
Answer: molar mass (grams to mole ratio) of H2 found on the periodic table
Step 10:
How do I set it up?
Answer: units first, set up the units that you need to cancel out (in red)
| 4 mol | 2 H2 | g = | g H2 |
| 1 | mol |
Step 11:
What is the next step?
Answer: Fill in numbers and cross out units
| 4 mol | 2 H2 | 2 g = | g H2 |
| 1 | 1 mol |
Step 12: Simplify
| 4 | 2 H2 | 2 g = | g H2 |
| 1 | 1 |
Step 13:
How do I know I am done with conversions?
Answer: The only units left are the units that match the answer. In this case g and H2
| 4 | 2 H2 | 2 g = | g H2 |
| 1 | 1 |
Step 14:
How do I do the calculations?
Answer: (4 * 2 * 2) = 16
| 4 | 2 H2 | 2 g = | 16 g H2 |
| 1 | 1 |
Step 15:
COMPLETE ANSWER: 16 g H2
VIDEO Stoichiometry Conversions Demonstrated Example 4: If you have 30g of CO2 then how many moles of C2H6 will be produced? You will need a periodic table to help solve this problem.
2 C2H6(l) + 7 O2(g) ——> 4 CO2(g) + 6 H2O(g)
Step 1:
What information does the question supply us with?
Answer: 30g CO2
Step 2:
What units does the question ask?
Answer: mol C2H6
Step 3:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 2 arrows when we go from Grams of A —> Moles of A —> Moles of B. 2 arrows = 2 conversions
Step 4:
How do we set up the problem?
Answer:
| 30 g CO2 | mol C2H6 | ||
| 1 |
Step 5:
What is the first conversion?
Answer: molar mass (grams to mole ratio) of CO2 found on the periodic table
Step 6:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 30 g CO2 | mol | mol C2H6 | |
| g |
Step 7:
What is the next step?
Answer: Fill in the numbers and cross out units
| 30 g CO2 | 1 mol | mol C2H6 | |
| 44 g |
Step 8: Simplify
| 30 CO2 | 1 mol | mol C2H6 | |
| 44 |
Step 9:
What is the next conversion?
Answer: mole to mole ratio (coefficient ratio)
Step 10:
How do I set it up?
Answer: units first, set up the units that you need to cancel out (in red)
| 30 CO2 | 1 mol | C2H6 | mol C2H6 |
| 44 | CO2 |
Step 11:
What is the next step?
Answer: Fill in numbers and cross out units
| 30 CO2 | 1 mol | 2 C2H6 | mol C2H6 |
| 44 | 4 CO2 |
Step 12: Simplify
| 30 | 1 mol | 2 C2H6 | mol C2H6 |
| 44 | 4 |
Step 13:
How do I know I am done with conversions?
Answer: The only units left are the units that match the answer. In this case mol and C2H6
| 30 | 1 mol | 2 C2H6 = | mol C2H6 |
| 44 | 4 |
Step 14:
How do I do the calculations?
Answer: (30 * 2) / (44 * 4) = 0.34
| 30 | 1 mol | 2 C2H6 = | 0.34 mol C2H6 |
| 44 | 4 |
Step 15:
COMPLETE ANSWER: 0.34 mol C2H6
VIDEO Stoichiometry Conversions Demonstrated Example 5: If you have 12.9 g of Ca3(PO4)2 then how many grams of NaCl will you need to completely react? You will need a periodic table to help solve this problem. You can also use the conversion map.
2 Na3PO4(aq) + 3 CaCl2(aq) ——> Ca3(PO4)2(s) + 6 NaCl(aq)
Step 1:
What information does the question supply us with?
Answer: 12.9 g Ca3(PO4)2
Step 2:
What units does the question ask?
Answer: g NaCl
Step 3:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 3 arrows when we go from Grams of A —> Moles of A —> Moles of B —> Grams of B. 3 arrows = 3 conversions
Step 4:
How do we set up the problem?
Answer:
| 12.9 g Ca3(PO4)2 | g NaCl | |||
| 1 |
Step 5:
What is the first conversion?
Answer: molar mass (grams to mole ratio) of Ca3(PO4)2 found on the periodic table
Step 6:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 12.9 g Ca3(PO4)2 | mol | g NaCl | ||
| g |
Step 7:
What is the next step?
Answer: Fill in the numbers and cross out units
| 12.9 g Ca3(PO4)2 | 1 mol | g NaCl | ||
| 310 g |
Step 8: Simplify
| 12.9 Ca3(PO4)2 | 1 mol | g NaCl | ||
| 310 |
Step 9:
What is the next conversion?
Answer: mole to mole ratio (coefficient ratio)
Step 10:
How do I set it up?
Answer: units first, set up the units that you need to cancel out (in red)
| 12.9 Ca3(PO4)2 | 1 mol | NaCl | g NaCl | |
| 310 | Ca3(PO4)2 |
Step 11:
What is the next step?
Answer: Fill in the numbers and cross out units
| 12.9 Ca3(PO4)2 | 1 mol | 6 NaCl | g NaCl | |
| 310 | 1 Ca3(PO4)2 |
Step 12: Simplify
| 12.9 | 1 mol | 6 NaCl | g NaCl | |
| 310 | 1 |
Step 13:
What is the next conversion?
Answer: molar mass (grams to mole ratio) of NaCl found on the periodic table
Step 14:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 12.9 | 1 mol | 6 NaCl | g = | g NaCl |
| 310 | 1 | mol |
Step 15:
What is the next step?
Answer: Fill in the numbers and cross out units
| 12.9 | 1 mol | 6 NaCl | 58 g = | g NaCl |
| 310 | 1 | 1 mol |
Step 16: Simplify
| 12.9 | 1 | 6 NaCl | 58 g = | g NaCl |
| 310 | 1 | 1 |
Step 17:
How do I know I am done with conversions?
Answer: The only units left are the units that match the answer. In this case g and NaCl
| 12.9 | 1 | 6 NaCl | 58 g = | g NaCl |
| 310 | 1 | 1 |
Step 18:
How do I do the calculations?
Answer: (12.9 * 6 * 58) / (310) = 14.5
| 12.9 | 1 | 6 NaCl | 58 g = | 14.5 g NaCl |
| 310 | 1 | 1 |
Step 19:
COMPLETE ANSWER: 14.5 g NaCl
PRACTICE PROBLEMS: Calculate the moles or grams you can obtain from the moles or grams you are given. You will need the periodic table.
If you have 3 moles of Mg then how many grams of O2 will you need?
2 Mg + O2 —> 2 MgO
Answer: 48 g O2
If 25 g of Ca(OH)2 are used, how many moles of NaOH can you make?
Ca(OH)2 + Na2S —> CaS + 2 NaOH
Answer: 0.68 mol NaOH
How many grams of Al do you need if you have 8 g of Br2?
2 AlBr3 —> 2 Al + 3 Br2
Answer: 0.9 g Al
If you have 0.6g of CO2, how many grams of O2 can you make?
2 C4H10 + 13 O2 —> 8 CO2 + 10 H2O
Answer: 0.71 g O2
Using Stoichiometry in Conversions
We can further expand our understanding of stoichiometry by using the system of ratios we have created as conversions for questions that we are asked in chemistry. This section builds on the skills you have practiced starting in the unit conversions section and continued in the grams moles molecules and atoms lesson. These questions may seem strange to some students, but we use them in everyday activities. Baking and cooking are the best example I can think of. All baking recipes have specific ratios of ingredients. For example, a batch of cookies might require 2 eggs and 3 cups of sugar for 24 cookies. However, if you want to make 48 cookies (double the recipe. Then you need 2 * 2 = 4 eggs and 2 * 3 = 6 cups of sugar. We can display this information in a chemical equation and a stoichiometric conversion.
2 eggs + 3 cups of sugar —> 24 cookies
| 48 cookies | 2 eggs | = 4 eggs |
| 24 cookies |
(48 * 2)/24 = 4
| 48 cookies | 3 cups of sugar | = 6 cups of sugar |
| 24 cookies |
(48 * 3)/24 = 6
In the same way we use this technique for baking or cooking, we can also use it for chemistry. This is because baking and cooking are chemistry. In chemical stoichiometry, the unit we most often use is the MOLE. So, any coefficient in a chemical equation can be used to describe the mole-to-mole relationship. The unit of the mole is the currency of chemistry, the same way the dollar is the currency of the United States. This means that all transactions in chemistry eventually have to be converted into moles. Just like all transactions in the United States eventually have to be translated into dollars. This means that the mole conversions for stoichiometry are the most important conversions to learn. The picture below is a representation of what I am saying. It can be used as a map for guiding you through these conversions. As you work your way through chemistry problems use the map to guide you. Every step you take or every arrow that you pass through means you have to do 1 conversion. Right now it is a very basic map that should be very straightforward, but as you get deeper into chemistry this map will grow more and more complex. Remember your problem solving skills. Write down your information first.
VIDEO Stoichiometry Conversions Demonstrated Example 1: How many moles of Mg can be made from 5 moles of Na given the chemical equation below?
2 Na + MgBr2 ——> Mg + 2 NaBr
Step 1:
What information does the question supply us with?
Answer: 5 mol Na
Step 2:
What units does the question ask?
Answer: mol Mg
Step 3:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 1 arrow when we go from moles of A —> moles of B. 1 arrows = 1 conversion
Step 4:
How do we set up the problem?
Answer:
| 5 mol Na | mol Mg | |
| 1 |
Step 5:
What is the first conversion?
Answer: mole to mole ratio
Step 6:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 5 mol Na | Mg = | mol Mg |
| Na |
Step 7:
What is the next step?
Answer: Fill in the numbers and cross out units
| 5 mol Na | 1 Mg = | mol Mg |
| 2 Na |
Step 8:
How do I know I am done with conversions?
Answer: The only units left are the units that match the answer. In this case mol and Mg
| 5 mol Na | 1 Mg = | mol Mg |
| 2 Na |
Step 9: Simplify
| 5 mol | 1 Mg = | mol Mg |
| 2 |
Step 10:
How do I do the calculations?
Answer: (5 * 1) / 2 = 2.5
Step 11:
What is the complete answer?
COMPLETE ANSWER: 2.5 mol Mg
VIDEO Stoichiometry Conversions Demonstrated Example 2: If you have 13.4 mol of Ca then how many moles of Ag3PO4 can you make?
6 Ag(s) + Ca3(PO4)2(s) ——> 3 Ca(s) + 2 Ag3PO4(s)
Step 1:
What information does the question supply us with?
Answer: 13.4 mol Ca
Step 2:
What units does the question ask?
Answer: mol Ag3PO4
Step 3:
How many conversions must we do?
Answer: Look at the conversion map. We pass through 1 arrow when we go from moles of A —> moles of B. 1 arrows = 1 conversion
Step 4:
How do we set up the problem?
Answer:
| 13.4 mol Ca | mol Ag3PO4 | |
| 1 |
Step 5:
What is the first conversion?
Answer: mole to mole ratio
Step 6:
How do I put that in?
Answer: units first, set up the units that need to cancel out (in red)
| 13.4 mol Ca | Ag3PO4 = | mol Ag3PO4 |
| Ca |
Step 7:
What is the next step?
Answer: Fill in the numbers and cross out units
| 13.4 mol Ca | 2 Ag3PO4 = | mol Ag3PO4 |
| 3 Ca |
Step 8: Simplify
| 13.4 mol | 2 Ag3PO4 = | mol Ag3PO4 |
| 3 |
Step 9:
How do I know I am done with conversions?
Answer: The only units left are the units that match the answer. In this case mol and Ag3PO4
| 13.4 mol | 2 Ag3PO4 = | mol Ag3PO4 |
| 3 |
Step 10:
How do I do the calculations?
Answer: (13.4 * 2) / 3 = 8.93
Step 11:
What is the complete answer?
COMPLETE ANSWER: 8.93 mol Ag3PO4
PRACTICE PROBLEMS: Calculate the moles you can obtain from the moles you are given. Use the conversion map table if you need it.
How many moles of BaCl2 can be made from 8 moles of HCl given the chemical equation below?
Ba(OH)2(aq) + 2 HCl(aq) ——> 2 H2O(l) + BaCl2(aq)
Answer: 8 mol HCl
How many moles of (NH4)3P can be made from 7 moles of NH4F given the chemical equation below?
3 NiF2(s) + 2 (NH4)3P(s) ——> 6 NH4F(aq) + Ni3P2(s)
Answer: 2.33 mol (NH4)3P
If you have 10 mol of MnI3 then how many moles of Mn can you make?
2 MnI3(s) ——> 3 I2(g) + Mn(s)
Answer: 5 mol Mn
If you have 4.2 mol of CO2 then how many moles of C4H10 can you make?
2 C4H10(l) + 13 O2(g) ——> 8 CO2(g) + 10 H2O (g)
Answer: 1.05 mol C4H10
How many moles of V3P2 are required to completely react with 0.63 moles of Ag given the chemical equation below?
2 Ag3P(s) + 3 V(s) ——> V3P2(s) + 6 Ag(s)
Answer: 0.105 mol V3P2
Chem – Stoichiometry Conversion Ratios
STOICHIOMETRY CONVERSION RATIOS (MOLE TO MOLE RATIOS):
Many students think stoichiometry is a scary and evil word, but it is just another type of conversion we see in chemistry. Stoichiometry is using the coefficients of a chemical equation to set up a conversion with them. Lets look at an example of what I am talking about with the chemical equation below. Ignore the states of the equation. They are not important in stoichiometry.
N2(g) + 3 H2(g) —-> 2 NH3(g)
From this chemical equation we can state some of the stoichiometry ratios:
What is the ratio between the N2 and the H2?
| 1 N2 |
| 3 H2 |
or
| 3 H2 |
| 1 N2 |
What is the ratio between the N2 and the NH3?
| 1 N2 |
| 2 NH3 |
or
| 2 NH3 |
| 1 N2 |
What is the ratio between the H2 and the NH3?
| 3 H2 |
| 2 NH3 |
or
| 2 NH3 |
| 3 H2 |
VIDEO Stoichiometry Ratios Demonstrated Example 1: For the chemical equation below what is the ratio of oxygen to H2?
H2SO4(aq) —> H2(g) + S(s) + 2 O2(g)
How do we set up the ratio?
Answer: O2 on top and H2 on bottom
| O2 |
| H2 |
What numbers do we put in front of each?
Answer: The coefficients of each in the chemical equation
COMPLETE ANSWER:
| 2 O2 |
| 1 H2 |
VIDEO Stoichiometry Ratios Demonstrated Example 2: For the chemical equation below what is the ratio of H2O to O2?
2 C5H7OH(l) + 13 O2(g) ——> 10 CO2(g) + 8 H2O(g)
How do we set up the ratio?
Answer: H2O on top and O2 on bottom
| H2O |
| O2 |
What numbers do we put in front of each?
Answer: The coefficients of each in the chemical equation
COMPLETE ANSWER:
| 8 H2O |
| 13 O2 |
PRACTICE PROBLEMS: Give the stoichiometry (coefficient) ratio for the questions below.
What is the ratio of Fe2O3 to FeP?
4 P + 2 Fe2O3 ——> 4 FeP + 3 O2
| 2 Fe2O3 |
| 4 FeP |
What is the ratio of Al2S3 to PbS2?
3 PbS2 + 4 AlPO4 ——> 2 Al2S3 + Pb3(PO4)4
| 2 Al2S3 |
| 3 PbS2 |
What is the ratio of MgO to KF?
MgO + 2 KF ——> MgF2 + K2O
| 1 MgO |
| 2 KF |
What is the ratio of LiNO3 to Ba?
2 Li + Ba(NO3)2 ——> Ba + 2 LiNO3
| 2 LiNO3 |
| 1 Ba |
Chem – LESSON 12: Stoichiometry
What is the lesson about?
This lesson is all about the different units you can convert between when using chemical equations. If you put so much gasoline in a car how many revolutions can you expect it to perform based on the volume of the engine or how many miles can you go? If you want to bake so many cookies, then how much butter will you need in grams? This lesson allows you to answer questions like that.
Why is it critical to understand?
Stoichiometry is used very widely in chemistry because so many times we have to convert back to simple units like moles in order to form and understanding of how chemical equations work or what we want to happen. However, the real power of stoichiometry lies in its ability to predict the future. We are an organism that puts a high price on the ability to predict the future and things like stoichiometry is one of our greatest tools. It helps us predict things like planning how to build a community. How much water will it need, how many kilograms of lumber for housing, how much fertilizer can we make for the plants. Fundamentally all these questions go back to chemistry stoichiometry. Try to keep that in mind as you are grinding through the more abstract examples and practice problems in this lesson.
What should you know before attempting this lesson?
If you have trouble in this lesson go back to sections on Unit Conversions, Calculating the Molar Mass of Compounds, and Grams to Moles Conversions.
New Learning Sections:
—> Stoichiometry Conversion Ratios
—> Using Stoichiometry in Conversions
—> Combining Stoichiometry and Molar Mass
Reference Pages:
—> Stoichiometry Conversion Map
Worksheets:
Chem – Empirical and Molecular Formula from Mass and Percent Part 5
When you compared the oxygen to the sulfur in a mole ratio, you came out with a nice, neat number like 3. This doesn’t usually happen, but because I wanted you to focus on the process and not the exact numbers, I made a simpler problem for you. However, if you are comparing mole ratios and you come out with a number like 2.98 or 3.05, then you can safely assume it rounds off to 3. If you happen to come out with a number like 5.45 or 5.52, then you can safely assume it rounds off to 5.5. This is a bit trickier to deal with, but I just want to give you an idea of the rounding. We will try a problem like this next. WARNING: some teachers will even expect you to round to a number like 1.33 or 1.66. I am very against teaching this to students because it can cause unnecessary confusion. It is very hard to determine if you are rounding correctly in a problem like that. Since empirical and molecular formulas are not a huge part of chemistry, I don’t try to emphasize them too much. This is not something that will come up in most chapters so if you are struggling with it get down the basics and then move on.
PRACTICE PROBLEMS: Solve the empirical and molecular problems below. Use this periodic table if needed.
What is the molecular formula of a compound that has a molar mass of 478.8 g/mol and is composed of 70% Iron and 30% Oxygen?
Answer: Fe6O9
Chem – Empirical Formula from Percent Part 4
How do you get the empirical formula from the percent of each element?
VIDEO Empirical and Molecular Formula Demonstrated Example 3: What is the empirical formula if the compound has a mass that is 60% oxygen and 40% sulfur? Use this periodic table if needed.
What is all the information we are given?
Answer:
60% Oxygen = 0.6
40% Sulfur = 0.4
For the next step we have to take a leap of imagination. Let us pretend that we have a small pile of this compound in front of us and we are weighing it. What mass is it going to be? The trick is we can start with any mass we want because we were given percentages in the original question. The easiest mass to pick is 100 grams total. Why? Because it makes the first set of calculations very simple. Take a look for yourself in the next steps.
What is the mass of oxygen if our total mass is 100g?
Answer: 0.6 * 100g = 60g
What is the mass of sulfur if our total mass is 100g?
Answer: 0.4 * 100g = 40g
To create the empirical formula, we need to know the masses of each element. The most important thing is how many moles of each element we have.
How many moles of oxygen do we have?
| 60g | 1 mol = | 3.75 mol |
| 16 g |
Answer: 3.75 mol O
How many moles of sulfur do we have?
| 40g | 1 mol = | 1.25 mol |
| 32 g |
Answer: 1.25 mol S
If we take both of our answers for the moles of each element, we can now compare the two elements in a compound. The best way to compare them is to divide them. Since an empirical formula is nothing more than a mole ratio between two or more elements, we can do the same with the moles that we have from our above answers.
What is the ratio of oxygen to sulfur?
| Oxygen = | 3.75 mol = | 3 |
| Sulfur | 1.25 mol | 1 |
Answer: 3
What does this ratio mean?
Answer: It means for every 3 oxygen, we have 1 sulfur.
What is the empirical formula?
COMPLETE ANSWER: SO3
PRACTICE PROBLEMS: Solve the empirical formula problems below. Use this periodic table if needed.
What is the empirical formula of a compound that is 14% hydrogen and 86% carbon?
Answer: CH2
What is the empirical formula of a compound that is 42.5% sodium, 44.5% carbon, and 13% nitrogen?
Answer: Na2C4N
Chem – Empirical Formula from Mass Part 3
How do you get the empirical formula from the mass of each element?
Other types of empirical and molecular formula problems require you to take the mass of each element and form them into an empirical formula. Remember the mass in grams can always be converted to the moles of a chemical or element if we know which chemical or element we are talking about. This conversion between mass and moles was taught in the previous section on how to convert between grams and moles.
Once we have the moles that means we have the amount of something and we can use that amount of each element to compare their ratio by dividing them (using mathematical division).
VIDEO Empirical and Molecular Formula Demonstrated Example 2: What is the empirical formula if a compound has 61 grams of magnesium 7 grams of nitrogen and 23 grams of phosphorus? Use this periodic table if needed.
How many moles of magnesium do we have?
| 61g | 1 mol = | 2.51 mol |
| 24.3 g |
Answer: 2.51 mol Mg
How many moles of nitrogen do we have?
| 7g | 1 mol = | 0.5 mol |
| 14 g |
Answer: 0.5 mol N
How many moles of phosphorus do we have?
| 23g | 1 mol = | 0.74 mol |
| 31 g |
Answer: 0.74 mol P
What is the ratio of magnesium to nitrogen?
| Magnesium = | 2.51 mol = | 5 |
| Nitrogen | 0.5 mol | 1 |
Answer: 5
What does that mean?
Answer: for every 5 magnesium there is 1 nitrogen.
What is the ratio of phosphorus to nitrogen?
| Phosphorus = | 0.75 mol = | 1.5 |
| Nitrogen | 0.5 mol | 1 |
Answer: 1.5
What does that mean?
Answer: for every 1.5 phosphorus there is 1 nitrogen
If phosphorus = 1.5 and nitrogen = 1 and magnesium = 5 how do we create the empirical formula?
Answer: Since the empirical formula has to be all whole numbers (another word for that is integers), we have to multiply all of them by a number until we get whole numbers. Focus on those that have decimals.
What is the lowest number I can multiply 1.5 by to get a whole number?
Answer: 2
If I multiply all the numbers by 2 what do I get?
Answer:
2 * 1.5 = 3 P
2 * 1 = 2 N
2 * 5 = 10 Mg
What is my empirical formula?
COMPLETE ANSWER: Mg10N2P3 (It does not matter what order you wright the elements in.)
PRACTICE PROBLEMS: Solve the empirical formula problems below. Use this periodic table if needed.
What is the empirical formula of a compound that is 97.5g potassium and 46.2g silicon?
Answer: K3Si2
Chem – Empirical and Molecular Formulas by Molar Mass Part 2
How is the empirical and molecular formula linked to the molar mass?
Another way that empirical and molecular formulas can be asked about is in terms of comparing their molar masses. Lets try that example we had before where the molecular formula was N4O10 and the empirical was N2O5. What is the molar mass of the molecular formula? It is approximately 216g/mol. What is the molar mass of the empirical formula? It is approximately 108g/mol. If we divide 216g/mol by 108g/mol what do we get? We get an answer of 2. How did we go from N4O10 to N2O5 before? We divided it by 2. This is not a coincidence. The same factor that is used in the subscripts of the molecular and empirical formulas is also used when it comes to their differences in molar mass. You can use this information to solve problems that involve molar masses and empirical and molecular formulas. Let us check out one style of these questions that I commonly see.
Examples: If the empirical formula is CH2, what is the molecular formula if the molar mass is 42 g/mol?
Answer: C3H6
If the empirical formula is Al2O3, what is the molecular formula if the molar mass is 408 g/mol?
Answer: Al8O12
VIDEO Empirical and Molecular Formula Demonstrated Example 1: If the empirical formula is Ca3P2, what is the molecular formula if the molar mass is 546 g/mol? Use this periodic table if needed.
Step 1:
What information do they tell us in the problem?
Answer:
empirical formula is Ca3P2…and its molar mass is 182 g/mol
molecular formula is ???…and its molar mass is 546 g/mol
Step 2:
What units, compounds, or information look similar or can we compare?
Answer:
182 g/mol and 546 g/mol
Step 3:
How do we compare them?
Answer:
Divide 546 g/mol by 182 g/mol = 3
Step 4:
What does that 3 represent?
Answer:
It is the number that we multiply all the subscripts of the empirical formula by.
Step 5:
What is the molecular formula?
COMPLETE ANSWER: Ca9P6
PRACTICE PROBLEMS: Solve the molecular formula problems.
If the empirical formula is CH2O, what is the molecular formula if the molar mass is 150 g/mol?
Answer: C5H10O5
If the empirical formula is Sn3N4, what is the molecular formula if the molar mass is 1239 g/mol?
Answer: Sn9N12
Chem – Empirical and Molecular Formula Explanation Part 1
What are empirical and molecular formulas?
VIDEO Explanation of empirical and molecular formulas
This part of the lesson is understanding what chemistry books call the empirical and molecular formula. In terms of definition, the empirical formula is the simplest ratio of atoms in a compound written in subscripts. The molecular formula is a true number of atoms in a compound written in subscripts. I am not really concerned that you know these definitions, and they might be very confusing to you at the moment. Instead, let us focus on some example and practice problems.
Examples: Give one possible molecular formula for the empirical formula.
| Empirical | Molecular |
| CH2 | C3H6 |
| N2O3 | N10O15 |
| Na3P | Na12P3 |
Notice when you multiply the subscripts of the empirical formula all by the same number, you get the molecular formula. In the first example, if you multiply all the subscripts by 3 you get the molecular formula shown.
PRACTICE PROBLEMS: Give one possible molecular formula for the empirical formula.
| Empirical | Molecular |
| NaCl | NaCl or Na2Cl2 or Na3Cl3… |
| P4O3 | P4O3 or P8O6 or P12O9… |
| CH | CH or C2H2 or C3H3… |
| Al2O3 | Al2O3 or Al4O6 or Al6O9… |
The empirical and molecular formula can sometimes be the same answer, but it is rare that you will see examples that follow this model.
Now lets try going in the other direction, where you have the molecular formula but not the empirical formula. Instead of multiplying, you are now dividing by what they call the greatest common divisor.
Examples: Give the empirical formula of the molecular formula
| Molecular | Empirical |
| N4O10 | N2O5 |
| Fe9Cu3 | Fe3Cu |
| Cr4Br12 | CrBr3 |
Compare the molecular formula with the empirical formula. What is the difference above? For the first example, if you divide both subscripts of the molecular formula by 2 then you get the empirical formula. So the secret to these is to find the greatest common divisor.
PRACTICE PROBLEMS: Give the empirical formula of the molecular formula.
| Molecular | Empirical |
| Ca6Mg10 | Ca3Mg5 |
| Si4C36 | SiC9 |
| Ni12Zn18 | Ni2Zn3 |
| H22C11O33 | H2CO3 |
| Ag11F5 | Ag11F5 |